Question

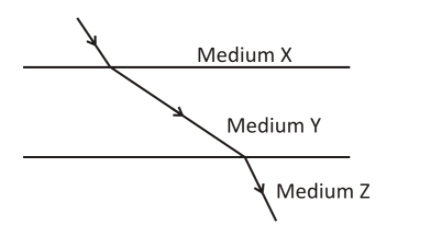
The refractive index of a medium 'X' with respect to 'Y' is $\dfrac{2}{3}$ and the refractive index of the medium ‘Y’ with respect to medium ‘Z’ is $\dfrac{4}{3}$. Calculate the refractive index of the medium ‘Z’ with respect to medium ‘X’.

Answer 1

Hint: The refractive index is defined as the ratio of velocity of light in vacuum or free space to the velocity of light in the given medium. Here we have to find the refractive index of a medium ‘X’ with respect to ‘Y’ and refractive index of a medium ‘Y’ with respect to ‘Z’.

Complete step by step solution:
Given:
Refractive index of a medium ‘X’ with respect to ‘Y’ i.e., ${N_{xy}} = \dfrac{2}{3}$
Refractive index of a medium ‘Y’ with respect to ‘Z’ i.e, ${N_{yz}} = \dfrac{4}{3}$

The refractive index is defined as the ratio of velocity of light in vacuum or free space to the velocity of light in the given medium and it is given by the formula:
\[{\text{Refractive index = }}\dfrac{{\left( {{\text{Velocity of light in vacuum}}} \right)}}{{\left( {{\text{Velocity of light in that medium}}} \right)}}\]
Refractive index of a medium ‘X’ with respect to ‘Y’ is:
${N_{XY}} = \dfrac{{{N_x}}}{{{N_y}}}$
Refractive index of a medium ‘Y’ with respect to ‘Z’ is:
 ${N_{yz}} = \dfrac{{{N_y}}}{{{N_z}}}$
The refractive index of ‘Z’ with respect to ‘X’ is given as:
${N_{zx}} = \dfrac{{{N_z}}}{{{N_x}}} = \dfrac{{{N_y}}}{{{N_x}}} = \dfrac{{{N_z}}}{{{N_y}}}$
\[\Rightarrow {\text{ }}{N_{zx}}{\text{ = }}\dfrac{1}{{{N_{xy}}}} \times \dfrac{1}{{{N_{yz}}}}\] …… (i)
On substituting the given values of ${N_{xy}} = \dfrac{2}{3}$ and ${N_{yz}} = \dfrac{4}{3}$ in equation (i) we get,
\[\therefore {\text{ }}{N_{zx}}{\text{ = }}\dfrac{9}{8}\]
So, the refractive index of medium ‘Z’ with respect to medium ‘X’ is equal to $\dfrac{9}{8}$.

Note: The phase velocity is the speed at which the crests of the wave move and can be faster than the speed of light in vacuum, and thereby give a refractive index below one. This can occur close to resonance frequencies, for absorbing media, in plasmas, and for X-rays.