
The relationship between mean, median and mode for a moderately skewed distribution is
A.
B.
C.
D.
Answer
437.1k+ views
1 likes
Hint: To find the relationship between mean, median and mode for a moderately skewed distribution, we should express this relationship by Karl Pearson’s formula. It is defined as the distance between the mean and the median is about one-third the distance between the mean and the mode. We can write this as
Complete step-by-step answer:
We need to find the relationship between mean, median and mode for a moderately skewed distribution. Let us see what skewed distribution is.

The above figure shows normal distribution. ‘A’ is known as the tail. In this distribution, we can see that
Let us see the types of skew distribution. There are 2 types –positive and negative.
Negatively-skewed distributions have a long left tail. They are also called negatively-skewed distributions. We can represent it as follows:
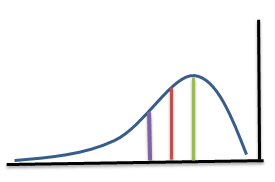
In the above figure, green colour indicates mode, red is median and violet is mean. From the figure, we can get the following relation
Now, let us see the positively-skewed distribution.
Positive-skew distributions have a long right tail. They are also called right-skewed distributions. We can represent it as follows:
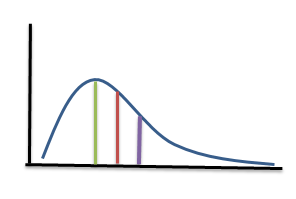
From the above figure, we can observe that
Now, let us see what moderately-skewed distribution is.
Highly skewed: Skewness is less than -1 or greater than 1
Moderately skewed: Skewness between -1 and -0.5 or between 0.5 and 1.
Approximately symmetric: Skewness is between -0.5 and 0.5.
We can express the relationship between mean, median and mode by Karl Pearson’s formula. It is defined as the distance between the mean and the median is about one-third the distance between the mean and the mode.
Let us now solve this. We will get
Let us now simplify the RHS. We will get
So, the correct answer is “Option D”.
Note: You may make an error when writing the formula
Complete step-by-step answer:
We need to find the relationship between mean, median and mode for a moderately skewed distribution. Let us see what skewed distribution is.

The above figure shows normal distribution. ‘A’ is known as the tail. In this distribution, we can see that
Let us see the types of skew distribution. There are 2 types –positive and negative.
Negatively-skewed distributions have a long left tail. They are also called negatively-skewed distributions. We can represent it as follows:

In the above figure, green colour indicates mode, red is median and violet is mean. From the figure, we can get the following relation
Now, let us see the positively-skewed distribution.
Positive-skew distributions have a long right tail. They are also called right-skewed distributions. We can represent it as follows:

From the above figure, we can observe that
Now, let us see what moderately-skewed distribution is.
Highly skewed: Skewness is less than -1 or greater than 1
Moderately skewed: Skewness between -1 and -0.5 or between 0.5 and 1.
Approximately symmetric: Skewness is between -0.5 and 0.5.
We can express the relationship between mean, median and mode by Karl Pearson’s formula. It is defined as the distance between the mean and the median is about one-third the distance between the mean and the mode.
Let us now solve this. We will get
Let us now simplify the RHS. We will get
So, the correct answer is “Option D”.
Note: You may make an error when writing the formula
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




