
The shadow of a tower standing on a level ground is found to be 60 metres longer
when the sun's altitude is
Answer
537k+ views
Hint:- Draw figure and then use trigonometry identity
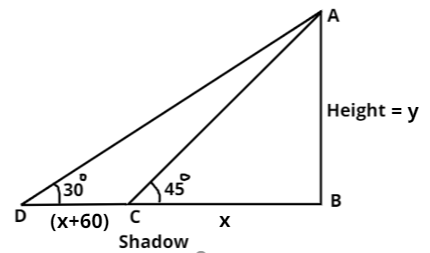
The above figure is drawn with respect to given conditions in question.
Let the height of the tower be AB
As we are given that the difference in length of shadows DC (see in figure) is 60 metres.
As, we can see from the above figure that the length of shadow when altitude
of sun is
So, length of the shadow when altitude of sun is
As we know that,
So, as we can see from the above figure in
So,
And in
Now, putting the value of
Now, as we have defined above that height of the tower is y metres.
Hence, the height of the tower will be 82 metres.
Note: - Whenever we come up with these types of problems then first, we should draw the figure according to the given conditions in question then, we will use the trigonometric functions to get the height of the tower, which will be the easiest and efficient method.

The above figure is drawn with respect to given conditions in question.
Let the height of the tower be AB
As we are given that the difference in length of shadows DC (see in figure) is 60 metres.
As, we can see from the above figure that the length of shadow when altitude
of sun is
So, length of the shadow when altitude of sun is
As we know that,
So, as we can see from the above figure in
So,
And in
Now, putting the value of
Now, as we have defined above that height of the tower is y metres.
Hence, the height of the tower will be 82 metres.
Note: - Whenever we come up with these types of problems then first, we should draw the figure according to the given conditions in question then, we will use the trigonometric functions to get the height of the tower, which will be the easiest and efficient method.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




