
The shape of the interference fringes, on the screen, is
A. Circle
B. Ellipse
C. Parabola
D. Straight line

Answer
488.1k+ views
Hint: In the figure, we have a mirror
Complete step by step answer:
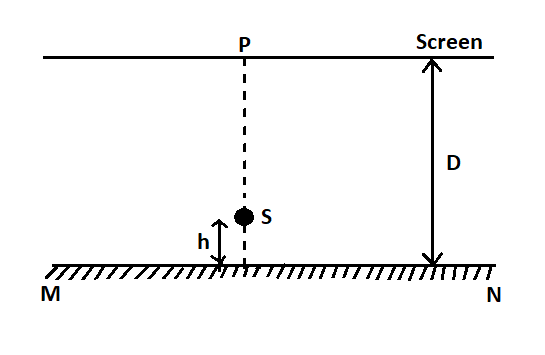
We have the setup of the mirror and the object given in the figure.
We know that we need two rays for the formation of an interference pattern.
Therefore, let us consider another point source as shown in the figure below.
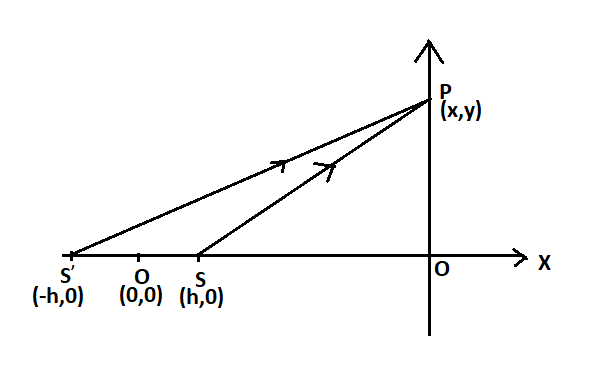
Let
The two rays
Let us consider that the two rays meet at a point
We can write the distance
We can write the distance
Let us take
The path difference can be written as,
From this we can write,
Squaring on both sides, we get
Substituting the values of
On solving we get
Cancelling the common terms and rearranging the equation we get,
Again squaring the equation,
Squaring we get
Expanding the equation we get,
Eliminating the common terms and rearranging the equation we get,
Making the LHS in terms of
This equation is of the form,
This is the equation for circle,
Hence fringes will appear circular.
So, the correct answer is “Option A”.
Note:
Light waves are considered electromagnetic waves. When two light waves of the same frequency overlap with each other interference happens and we get a pattern on the screen. The effect of the resultant pattern will depend on the amplitude of waves as well as the phases of the two waves. The resultant wave of interference is explained by the principle of superposition.
Complete step by step answer:
We have the setup of the mirror and the object given in the figure.
We know that we need two rays for the formation of an interference pattern.
Therefore, let us consider another point source as shown in the figure below.

Let
The two rays
Let us consider that the two rays meet at a point
We can write the distance
We can write the distance
Let us take
The path difference can be written as,
From this we can write,
Squaring on both sides, we get
Substituting the values of
On solving we get
Cancelling the common terms and rearranging the equation we get,
Again squaring the equation,
Squaring we get
Expanding the equation we get,
Eliminating the common terms and rearranging the equation we get,
Making the LHS in terms of
This equation is of the form,
This is the equation for circle,
Hence fringes will appear circular.
So, the correct answer is “Option A”.
Note:
Light waves are considered electromagnetic waves. When two light waves of the same frequency overlap with each other interference happens and we get a pattern on the screen. The effect of the resultant pattern will depend on the amplitude of waves as well as the phases of the two waves. The resultant wave of interference is explained by the principle of superposition.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




