
The slope of the graph between
(A)
(B)
(C)
(D)
Answer
438.6k+ views
2 likes
Hint :We know that according to Boyle’s law, for a given mass of a gas, the pressure is inversely proportional to volume occupied by that gas at a constant temperature. From this information, a relationship between
Complete Step By Step Answer:
According to Boyle’s law:
We can say that,
Taking logarithms of both sides, we get:
Applying the formula,
Rearranging the above equation:
Here,
Comparing the above equation with
Here,
Therefore, the slope of the graph between
The following slope graph is given as:
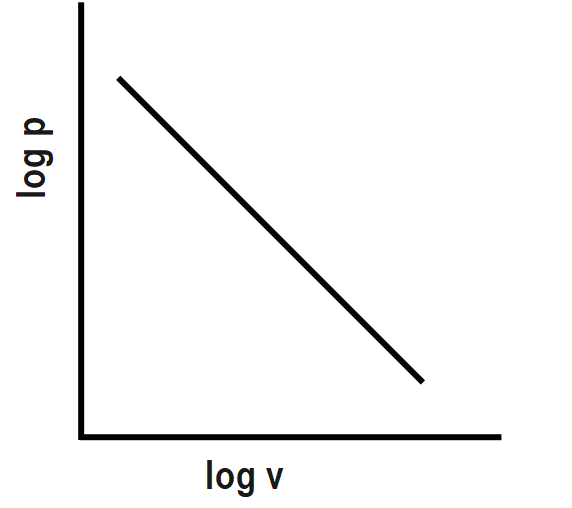
Hence, option (B) is the correct answer.
Note :
Remember that Boyle’s law holds good for an ideal gas. Gases are at high temperature and low pressures behave near to ideal gas. Here, we have assumed that the given gas behaves as an ideal gas. Therefore, the required slope can also be found out by using the ideal gas equation,
Taking logarithms of both sides, we get:
Here,
Rearranging the above equation:
Hence, we get the same value of slope,
Complete Step By Step Answer:
According to Boyle’s law:
We can say that,
Taking logarithms of both sides, we get:
Applying the formula,
Rearranging the above equation:
Here,
Comparing the above equation with
Here,
Therefore, the slope of the graph between
The following slope graph is given as:

Hence, option (B) is the correct answer.
Note :
Remember that Boyle’s law holds good for an ideal gas. Gases are at high temperature and low pressures behave near to ideal gas. Here, we have assumed that the given gas behaves as an ideal gas. Therefore, the required slope can also be found out by using the ideal gas equation,
Taking logarithms of both sides, we get:
Here,
Rearranging the above equation:
Hence, we get the same value of slope,
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




