
The sphere rolls down on an inclined plane of inclination
Answer
514.8k+ views
2 likes
Hint – In this question first draw a free body diagram of a sphere of radius R units and mass m. The mg will be acting vertically downwards from the sphere, so resolve this force with its two components that is
Complete step-by-step answer:
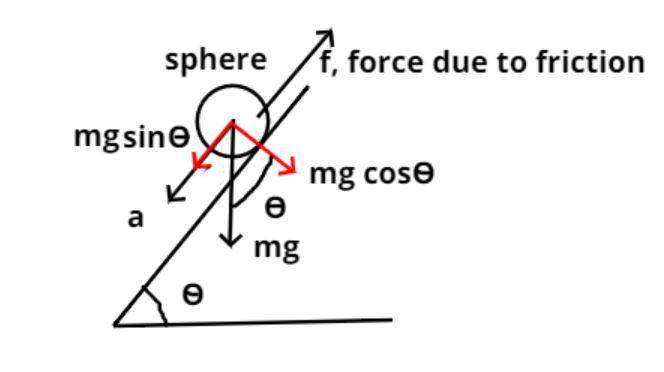
Sphere is rolling on the surface which is inclined at an angle
Let the radius of the sphere be R units.
Let the weight of the sphere be m kg.
So the force acting downward according to Newton’s second law of motion due to acceleration of the gravity (g) is given as
Let the acceleration of the sphere be (a) as shown in the figure.
So the force working on the sphere which is opposite to the direction of the movement according to Newton’s law is
Now the force
Let the force due to friction be (f) working opposite to the direction of movement of the sphere.
Now, resolve the component of force in
Now balance the forces we have,
Now substitute the value we have,
Now let the angular velocity and moment of inertia of the sphere be
So force due to friction (f) multiplied by the radius (R) is equal to the product of moment of inertia and angular velocity of the sphere.
Now the acceleration working on the sphere is the product of the radius of the sphere and the angular velocity of the sphere.
Now from equation (2) and (3) we have,
Now from equation (1) and (4) we have,
Now simplify this equation we have,
Now as we know that the moment of inertia of the sphere is
So substitute this value in above equation we have,
Now simplify this we have,
So this is the required acceleration working on the sphere.
Hence option (A) is the correct answer.
Note – The trick point here was regarding the concept of moment of inertia, a body always has a tendency to resist the angular acceleration so the entity that expresses this tendency is the moment of inertia. This is the physical significance of MOI. In general the moment of inertia is defined as the sum of the products of masses with the square of its distance from the axis of the rotation that is
Complete step-by-step answer:

Sphere is rolling on the surface which is inclined at an angle
Let the radius of the sphere be R units.
Let the weight of the sphere be m kg.
So the force acting downward according to Newton’s second law of motion due to acceleration of the gravity (g) is given as
Let the acceleration of the sphere be (a) as shown in the figure.
So the force working on the sphere which is opposite to the direction of the movement according to Newton’s law is
Now the force
Let the force due to friction be (f) working opposite to the direction of movement of the sphere.
Now, resolve the component of force in
Now balance the forces we have,
Now substitute the value we have,
Now let the angular velocity and moment of inertia of the sphere be
So force due to friction (f) multiplied by the radius (R) is equal to the product of moment of inertia and angular velocity of the sphere.
Now the acceleration working on the sphere is the product of the radius of the sphere and the angular velocity of the sphere.
Now from equation (2) and (3) we have,
Now from equation (1) and (4) we have,
Now simplify this equation we have,
Now as we know that the moment of inertia of the sphere is
So substitute this value in above equation we have,
Now simplify this we have,
So this is the required acceleration working on the sphere.
Hence option (A) is the correct answer.
Note – The trick point here was regarding the concept of moment of inertia, a body always has a tendency to resist the angular acceleration so the entity that expresses this tendency is the moment of inertia. This is the physical significance of MOI. In general the moment of inertia is defined as the sum of the products of masses with the square of its distance from the axis of the rotation that is
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




