Question

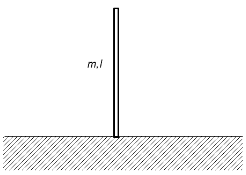
The thin rod is released from the vertical position as shown and falls by itself, then the angular speed of the rod just before it falls flat, will be

A) $\sqrt {\dfrac{g}{l}} $
B) $\sqrt {\dfrac{{3g}}{l}} $
C) $\sqrt {\dfrac{{4g}}{l}} $
D) $\sqrt {\dfrac{{6g}}{l}} $

Answer 1

Hint Calculate the potential energy of the rod by assuming it as a point particle having a mass equal to the mass of the rod and positioned at a height of the center of mass of the rod. Equalize the potential energy of the rod when it is upright with the rotational energy of the rod as it touches the ground.

Formula used: $U = mgh$ where $U$ is the potential energy of an object of mass $m$ at a height $h$ above the ground due to gravitational acceleration $g$.
${K_{rot}} = \dfrac{1}{2}I{\omega ^2}$ where ${K_{rot}}$ is the rotational kinetic energy of an object with moment of inertia $I$ and angular velocity $\omega $.

Complete step by step answer
To solve such problems, we can use the law of conservation of energy which tells us that the net energy of an object will remain conserved in a closed system. Since our surface is smooth, there will be no frictional energy loss in the system.
When the rod is at its vertical position, all its energy will be stored in the form of potential energy. And when it falls flat on the ground, the rod will only have rotational energy and no potential energy.
Now let’s calculate the potential energy of the rod when it is standing upright. The center of mass of the rod will be at the center of the rod at a height of $l/2$ above the ground since it has a uniform mass distribution. The potential energy of the rod can then be calculated assuming a point object of mass $m$ at a height $l/2$ above the ground as:
$\Rightarrow U = mg\dfrac{l}{2}$
When the rod falls flat on the ground, the center of mass of the rod will also be on the ground. The rod will have zero potential energy but it will have rotational kinetic energy associated with its angular speed.
From the law of conservation of energy, we can write
$\Rightarrow mg\dfrac{l}{2} = \dfrac{1}{2}I{\omega ^2}$
We know that the moment of inertia of a rod as it rotates about one of its end is given as
$\Rightarrow I = \dfrac{{m{l^2}}}{3}$
Substituting the value of the $I$, we get
$\Rightarrow mg\dfrac{l}{2} = \dfrac{1}{2}\dfrac{{m{l^2}}}{3}{\omega ^2}$
Dividing both sides by $\dfrac{{m{l^2}}}{2}$, we get
$\Rightarrow {\omega ^2} = \dfrac{{3g}}{l}$
Taking the square on both sides, we can calculate the angular speed as:
$\therefore \omega = \dfrac{{\sqrt {3g} }}{l}$ which corresponds to option (B).

Note
Since we’ve been given that the surfaces are smooth in the question; we are then free to use the law of conservation of energy. We then only have to deal with the initial state of the rod where all the energy is stored in the potential energy and the final state where the energy is in the form of kinetic energy. As a result, we don’t have to track the motion of the rod in between these two positions.