
The tops of two poles of height 16 m and 10 m are connected by a wire of length
(a) 26 m
(b) 16 m
(c) 12 m
(d) 10 m
Answer
521.4k+ views
Hint: For solving this problem first we will draw the geometrical figure as per the given data. After that, we will use the basic formula of trigonometry
Complete step-by-step answer:
Given:
It is given that the tops of two poles of height 16 m and 10 m are connected by a wire of length
Now, first, we will draw a geometrical figure as per the given data. For more clarity look at the figure given below:
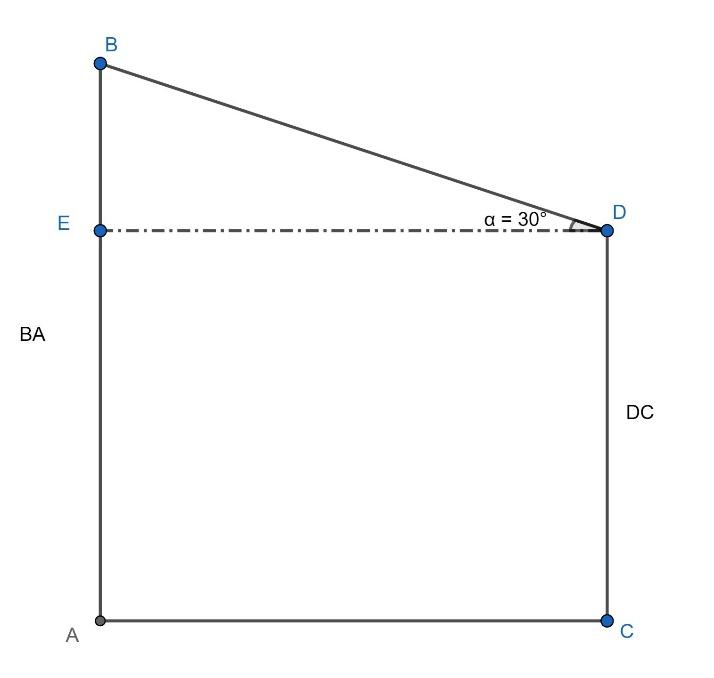
In the above figure BA is the pole of length 16 m, DC is the pole of length 10 m, BD is equal to the length of the wire
Now, as BA and DC are vertical as the poles will be standing vertical to the ground so, length EA will be equal to the length of the DC. Then,
Now, put the value of BA = 16 m and DC = 10 m in the above equation. Then,
Now, from the above result, we can write that the length of BE will be 6 m.
Now, we consider
Now, from the above result, we conclude that the length of BD will be 12 m.
Thus, the length of the wire
Hence, (c) is the correct option.
Note: Here, the student should first try to understand what is asked in the problem. After that, we should try to draw the geometrical figure as per the given data. Moreover, we should apply the basic formula of trigonometry properly without any error and avoid calculation mistakes while solving to get the correct answer.
Complete step-by-step answer:
Given:
It is given that the tops of two poles of height 16 m and 10 m are connected by a wire of length
Now, first, we will draw a geometrical figure as per the given data. For more clarity look at the figure given below:

In the above figure BA is the pole of length 16 m, DC is the pole of length 10 m, BD is equal to the length of the wire
Now, as BA and DC are vertical as the poles will be standing vertical to the ground so, length EA will be equal to the length of the DC. Then,
Now, put the value of BA = 16 m and DC = 10 m in the above equation. Then,
Now, from the above result, we can write that the length of BE will be 6 m.
Now, we consider
Now, from the above result, we conclude that the length of BD will be 12 m.
Thus, the length of the wire
Hence, (c) is the correct option.
Note: Here, the student should first try to understand what is asked in the problem. After that, we should try to draw the geometrical figure as per the given data. Moreover, we should apply the basic formula of trigonometry properly without any error and avoid calculation mistakes while solving to get the correct answer.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




