Question

The total flux associated with the given cube will be

Where \['a'\] is the side of the cube, \[\left( {\dfrac{1}{{{\varepsilon _0}}} = 4\pi \times 9 \times {{10}^9}SI\,unit} \right)\]

$ \left( A \right)162\pi \times {10^{ - 3}}N{m^2}{C^{ - 1}} \\$
$ \left( B \right)162\pi \times {10^3}N{m^2}{C^{ - 1}} \\$
$ \left( C \right)162\pi \times {10^{ - 6}}N{m^2}{C^{ - 1}} \\$
$ \left( D \right)162\pi \times {10^6}N{m^2}{C^{ - 1}} \\ $

Answer 1

Hint: In order to solve this question, we are going to firstly write the formula for the flux associated with any Gaussian surface. After that, taking this case of the cube, we will put the values of the charge and then calculate the flux for the given cube as shown in the figure.

Formula used: The total flux associated with any Gaussian surface is given by:
\[Total\,flux = \dfrac{{{q_{in}}}}{{{\varepsilon _0}}}\]
Where \[{q_{in}}\] is the charge contained in the given cube, \[{\varepsilon _0}\] is the permittivity of the free space.


Complete answer:
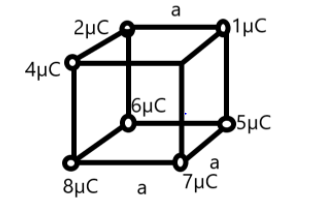
In the given figure, we can see a cube that is constrained in the three coordinates. If we look at the figure carefully, we see that the cube acts as a Gaussian surface. Now, we know that the total flux associated with any Gaussian surface is given by:
\[Total\,flux = \dfrac{{{q_{in}}}}{{{\varepsilon _0}}}\]
Where \[{q_{in}}\] is the charge contained in the given cube, \[{\varepsilon _0}\] is the permittivity of the free space.
In the given figure, the charge in the cube is shared by the eight corners, so, the charge is equal to \[\dfrac{1}{8}\] of the total charge is inside the cube.
Where, \[{q_{in}} = 1\mu + 2\mu + 3\mu + 4\mu + 5\mu + 6\mu + 7\mu + 8\mu = 36\mu \]
This implies that
The flux for the given cube is:
\[\phi = \dfrac{{{q_{in}}}}{{{\varepsilon _0}}} = \dfrac{{36\mu }}{{8{\varepsilon _0}}}\]
Putting the values in this, we get
\[\phi = \dfrac{{36}}{8} \times {10^{ - 6}} \times 4\pi \times 9 \times {10^9} = 162\pi \times {10^3}\]
Hence, option \[\left( B \right)162\pi \times {10^3}N{m^2}{C^{ - 1}}\] is the correct answer.

Note: The equation for the flux through any Gaussian surface has been deduced from the Gauss’s law. According to Gauss's law, the flux of the electric field through any closed surface, also called a Gaussian surface, is equal to the net charge enclosed divided by the permittivity of free space.