
The two adjacent sides of a cyclic quadrilateral are 2 and 5 and the angle between them is
Answer
501.3k+ views
Hint: Draw a diagram of the given cyclic quadrilateral and assign all the given data in the question. Use the law of sine and cosine rule to find out the length of other sides.
Complete step by step answer:
We know that the cyclic quadrilateral is inscribed within a circle or the vertices of a cyclic quadrilateral lies on a circle. We also know that the sum of opposite angles in a quadrilateral is
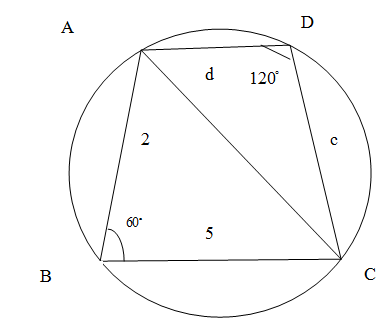
We draw diagram of the given cyclic quadrilateral
We know from the sine rule that area of a triangle with lengths with two sides
Putting in equation(1)
Similarly the area of the triangle
It is also given that the area of the quadrilateral
We also know from the cosine rule that the relation in the triangle
Similarly in the triangle
So we equate right hand side of equation (2) and (3) and put known values
We obtained that
Now
So the length of other two sides are 2 and 3.
Note: The question tests your knowledge of cyclic quadrilateral, sine rule and cosine rule. Careful solving of simultaneous equations with substitution and usage of formula will lead us to arrive at the correct result. The question can also be reframed to find the rest of the angles.
Complete step by step answer:
We know that the cyclic quadrilateral is inscribed within a circle or the vertices of a cyclic quadrilateral lies on a circle. We also know that the sum of opposite angles in a quadrilateral is

We draw diagram of the given cyclic quadrilateral
We know from the sine rule that area of a triangle with lengths with two sides
Putting in equation(1)
Similarly the area of the triangle
It is also given that the area of the quadrilateral
We also know from the cosine rule that the relation in the triangle
Similarly in the triangle
So we equate right hand side of equation (2) and (3) and put known values
We obtained that
Now
So the length of other two sides are 2 and 3.
Note: The question tests your knowledge of cyclic quadrilateral, sine rule and cosine rule. Careful solving of simultaneous equations with substitution and usage of formula will lead us to arrive at the correct result. The question can also be reframed to find the rest of the angles.
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




