Question

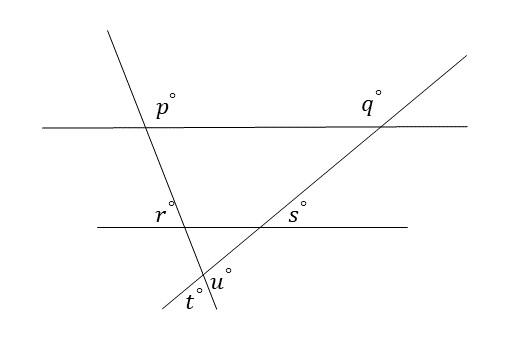
The two horizontal lines shown in the above figure are parallel to each other. Which of the following does NOT equal ${180^\circ }$.

\[
  \left( A \right)\quad {\left( {p + r} \right)^\circ } \\
  \left( B \right)\quad {\left( {p + t} \right)^\circ } \\
  \left( C \right)\quad {\left( {q + s} \right)^\circ } \\
  \left( D \right)\quad {\left( {r + s + t} \right)^\circ } \\
  \left( E \right)\quad {\left( {t + u} \right)^\circ } \\
 \]

Answer 1

Hint: We will be solving the question by individually checking the options provided to us. We will use the properties of angles such as
$\left( 1 \right)$ Sum of Supplementary angles is ${180^\circ }$.
$\left( 2 \right)$ Sum of all interior angles of a triangle is ${180^\circ }$.
$\left( 3 \right)$ Vertical angles are equal.
$\left( 4 \right)$ Corresponding angles are equal.

Complete step-by-step answer:
Let us add some more angles in the figure in order to understand better
Checking option \[\left( A \right)\quad {\left( {p + r} \right)^\circ }\]
As we can observe that
$\angle p + \angle m = {180^\circ }$ (Supplementary angles)
And $\angle m = \angle r$ (Corresponding angles)
$ \Rightarrow \angle p + \angle m = \angle p + \angle r = {180^\circ }$ (Since they are equal)

Checking option \[\left( B \right)\quad {\left( {p + t} \right)^\circ }\]
We can see that from the data given we cannot conclude that the value of \[{\left( {p + t} \right)^\circ } = {180^\circ }\].
Therefore, we will check other options.
Checking option \[\left( C \right)\quad {\left( {q + s} \right)^\circ }\]
As we can observe that
$\angle q + \angle n = {180^\circ }$ (Supplementary angles)
And $\angle n = \angle s$ (Corresponding angles)
$ \Rightarrow \angle q + \angle n = \angle q + \angle s = {180^\circ }$ (Since they are equal)
Checking option \[\left( D \right)\quad {\left( {r + s + t} \right)^\circ }\]
As we can observe from the figure
$
  \angle r = \angle x \\
  \angle s = \angle y \\
  \angle t = \angle z \\
 $(Vertical angles)
In addition, we know that sum of all interior angles of a triangle $ = {180^\circ }$.Therefore,
$
   \Rightarrow \angle x + \angle y + \angle z = {180^\circ } \\
   \Rightarrow \angle r + \angle s + \angle t = {180^\circ } \\
 $
Therefore \[{\left( {r + s + t} \right)^\circ } = 180^\circ \]
Checking option \[\left( E \right)\quad {\left( {t + u} \right)^\circ }\]
We can see that $\angle t$ and $\angle u$ are supplementary angles. Therefore,
$ \Rightarrow \angle t + \angle u = 180^\circ $
After checking all the options, we can Conclude that the options $\left( A \right),\left( C \right),\left( D \right),\left( E \right)$ are all equal to ${180^\circ }$. So by eliminating these options, we are only left with option $\left( B \right)$
Hence, the correct answer is $\left( B \right)$.

Note: It should be noted that the angles $r,s\;and\;t$ are not the exterior angles of the triangle formed. Therefore, you cannot apply “the sum of exterior angles of a convex polygon is ${360^0}$” property.