
The upper half of an inclined plane of inclination
Answer
513.9k+ views
Hint: Apply Newton’s law of motion separately for both the motions. Final velocity of the first half will be equal to initial velocity of the second half. Magnitude of displacement for both the motions is the same. As we are not asked about time, Newton’s third law of motion will be helpful.
Complete Step By Step Solution:
Let us assume mass of block be m kg, g be acceleration due to gravity, total length of incline l and velocity at halfway v. free body diagram of the block for first half,
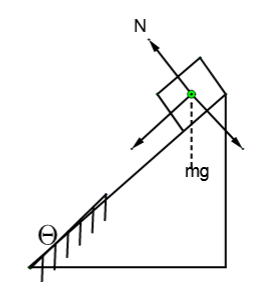
The acceleration of block will be along the incline and equal to
As the acceleration is constant for the motion we can apply Newton’s law of motion
Applying Newton’s third law of motion form starting to before reaching rough surface
Now, free body diagram of the block for second half
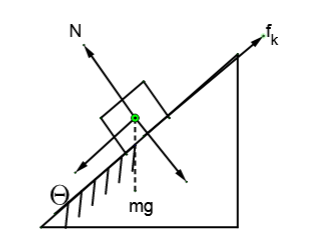
Here as the block is moving only along the incline, net force perpendicular to it must be zero. So,
Also the block is sliding over incline, so friction will be
Acceleration of block will b along the incline will be
As the acceleration is constant for the motion we can apply Newton’s law of motion
Applying Newton’s third law of motion form starting of rough surface to bottom
Putting value of
Hence the correct option is A.
Note: This question can be solved with the following method. We know that both half motions are almost identical as initial velocity and final velocity has just got interchanged and length of both motions is the same too. So, we can say the magnitude of acceleration of both should be the same and the direction of acceleration of both should be opposite. So, we will get
Complete Step By Step Solution:
Let us assume mass of block be m kg, g be acceleration due to gravity, total length of incline l and velocity at halfway v. free body diagram of the block for first half,

The acceleration of block will be along the incline and equal to
As the acceleration is constant for the motion we can apply Newton’s law of motion
Applying Newton’s third law of motion form starting to before reaching rough surface
Now, free body diagram of the block for second half

Here as the block is moving only along the incline, net force perpendicular to it must be zero. So,
Also the block is sliding over incline, so friction will be
Acceleration of block will b along the incline will be
As the acceleration is constant for the motion we can apply Newton’s law of motion
Applying Newton’s third law of motion form starting of rough surface to bottom
Putting value of
Hence the correct option is A.
Note: This question can be solved with the following method. We know that both half motions are almost identical as initial velocity and final velocity has just got interchanged and length of both motions is the same too. So, we can say the magnitude of acceleration of both should be the same and the direction of acceleration of both should be opposite. So, we will get
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




