
The value of g at a certain height h above the free surface of the earth is
A. R
B. 2R
C. 3R
D. 4R
Answer
520.2k+ views
Hint: In this question, we first start by writing the general expression of g that is
Complete Step-by-Step solution:
We all know that the acceleration due to gravity is denoted by the symbol g and is defined as the acceleration of an object because of the force acting on that object by the gravitational field of Earth.
So we start by writing the expression for acceleration due to gravity g when an object of mass m is placed at a distance r from the center of the earth with mass M and radius R is given as:
Now in this question, it is given that the acceleration due to gravity g when the object is on the surface of the earth that is when
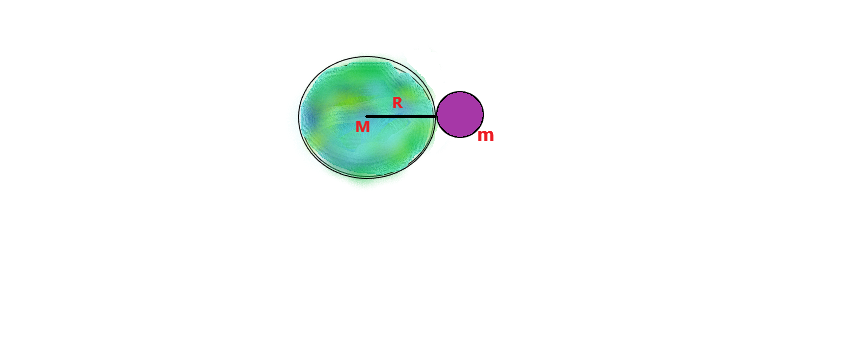
So we can write the expression for
Also, it is given that the acceleration due to gravity g when the object is placed at a height h above the surface of the earth that is when
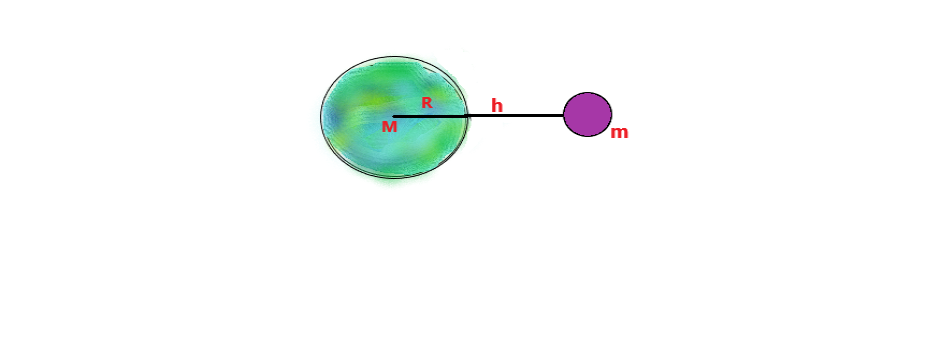
Similarly we can write the expression for
Now we take
Now using equation (1) we can replace
Taking square root on both sides we will get
Taking
Hence the height h is equal to R that is the radius of the earth. So option A is correct.
Note: For these types of questions we need to have a clear understanding of how the acceleration due to gravity varies with the position of the object from the center of the earth that is
Complete Step-by-Step solution:
We all know that the acceleration due to gravity is denoted by the symbol g and is defined as the acceleration of an object because of the force acting on that object by the gravitational field of Earth.
So we start by writing the expression for acceleration due to gravity g when an object of mass m is placed at a distance r from the center of the earth with mass M and radius R is given as:
Now in this question, it is given that the acceleration due to gravity g when the object is on the surface of the earth that is when

Figure 1
So we can write the expression for
Also, it is given that the acceleration due to gravity g when the object is placed at a height h above the surface of the earth that is when

Figure 2
Similarly we can write the expression for
Now we take
Now using equation (1) we can replace
Taking square root on both sides we will get
Taking
Hence the height h is equal to R that is the radius of the earth. So option A is correct.
Note: For these types of questions we need to have a clear understanding of how the acceleration due to gravity varies with the position of the object from the center of the earth that is
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Discuss the main reasons for poverty in India




