-
Courses-
Crash Courses 2021
-
Olympiads/NTSE/Others
-
Micro Courses
-
Vedantu Super Kids NEW
-
Reading & Spoken English -
FREEMaster Classes
-
PREMIUM1-on-1 Classes
-
Study Material
-
NCERT Solutions
-
Previous Year Papers
-
Mock Tests
-
Sample Papers
-
Reference Book Solutions
-
ICSE Solutions
-
School Syllabus
-
Revision Notes
-
Important Questions
-
Math Formula Sheets
-
-
More
Book your FREE Online counselling session
Share your contact information
Your FREE Online counselling session has been booked!
Vedantu academic counsellor will be calling you shortly for your Online Counselling session.
DONE
Please try again later
OK
Questions & Answers
Question
Answers
Related Questions
The values of \[\alpha \] if \[(\alpha ,2\alpha )\]lies inside the \[\Delta ABC\] if
\[A(0,2),B(2,0)\]and \[C(4,4)\]
(a) \[\alpha \in \left( \dfrac{1}{3},\dfrac{2}{3} \right)\]
(b) \[\alpha \in \left( \dfrac{2}{3},1 \right)\]
(c) \[\alpha \in \left( \dfrac{2}{3},\dfrac{4}{3} \right)\]
(d) \[\alpha \in \left( \dfrac{1}{3},1 \right)\]
Answer
![Verified]()
Verified
Verified
Hint: Find the equation of the lines which are forming the triangle.
The figure for the given problem is as follows:
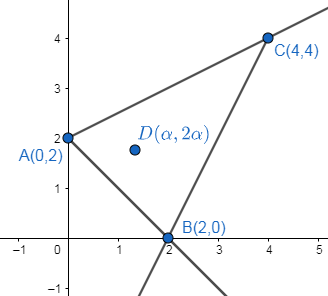
Now we will find the equations of all the three sides of the triangle.
We know equation of line between the two points \[({{x}_{1}},{{y}_{1}})\] and \[({{x}_{2}},{{y}_{2}})\]can be written as,
\[\dfrac{y-{{y}_{1}}}{{{y}_{2}}-{{y}_{1}}}=\dfrac{x-{{x}_{1}}}{{{x}_{2}}-{{x}_{1}}}\]
Applying the above formula, the equation of side AB is,
\[\dfrac{y-2}{0-2}=\dfrac{x-0}{2-0}\]
\[\dfrac{y-2}{-2}=\dfrac{x}{2}\]
\[\dfrac{y-2}{-1}=\dfrac{x}{1}\]
On cross multiplication, we get
\[y-2=-x\]
\[x+y-2=0........(i)\]
Similarly, the equation of side BC is,
\[\dfrac{y-0}{4-0}=\dfrac{x-2}{4-2}\]
\[\dfrac{y}{4}=\dfrac{x-2}{2}\]
\[\dfrac{y}{2}=\dfrac{x-2}{1}\]
On cross multiplication, we get
\[y=2x-4\]
\[2x-y-4=0........(ii)\]
And, the equation of side AC is,
\[\dfrac{y-2}{4-2}=\dfrac{x-0}{4-0}\]
\[\dfrac{y-2}{2}=\dfrac{x}{4}\]
\[\dfrac{y-2}{1}=\dfrac{x}{2}\]
On cross multiplication, we get
\[2y-4=x\]
\[x-2y+4=0........(iii)\]
Therefore the figure with equations is,
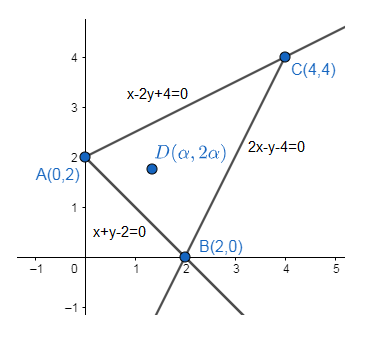
Two given points \[\left( {{x}_{1}},\text{ }{{y}_{1}} \right)\]and \[\left( {{x}_{2}},\text{ }{{y}_{2}} \right)\]will lie on the same side of the line \[ax+by+c=0\] if \[a{{x}_{1}}+b{{y}_{1}}+c\] and \[a{{x}_{2}}+b{{y}_{2}}+c\] will have same signs.
From the above figure it is clear that the points B and D lie on the same side of the line AC. So, it should satisfy the above condition, i.e.,
\[2-2(0)+4=6>0\]
So, when we substitute the value of point D in line AC, it should be greater than zero, i.e.,
\[\alpha -2(2\alpha )+4>0\]
\[\alpha -4\alpha +4>0\]
\[-3\alpha +4>0\]
\[4>3\alpha \]
\[\Rightarrow \alpha <\dfrac{4}{3}.........(iv)\]
Now from the above figure it is clear that the points C and D lie on the same side of the line AB. Substitute value of point C in equation of line AB, we get
\[4+4-2=6>0\]
So, when we substitute the value of point D in line AB, it should be greater than zero, i.e.,
\[\alpha +2\alpha -2>0\]
\[3\alpha -2>0\]
\[3\alpha >2\]
\[\Rightarrow \alpha >\dfrac{2}{3}.........(v)\]
So, from equation (iv) and (v), we get
\[\alpha \in \left( \dfrac{2}{3},\dfrac{4}{3} \right)\]
Hence the correct answer is option (c).
Note: We can solve this by finding boundaries of x and y. But the options are given in fraction form, using this method it gives the exact answer.
The figure for the given problem is as follows:
Now we will find the equations of all the three sides of the triangle.
We know equation of line between the two points \[({{x}_{1}},{{y}_{1}})\] and \[({{x}_{2}},{{y}_{2}})\]can be written as,
\[\dfrac{y-{{y}_{1}}}{{{y}_{2}}-{{y}_{1}}}=\dfrac{x-{{x}_{1}}}{{{x}_{2}}-{{x}_{1}}}\]
Applying the above formula, the equation of side AB is,
\[\dfrac{y-2}{0-2}=\dfrac{x-0}{2-0}\]
\[\dfrac{y-2}{-2}=\dfrac{x}{2}\]
\[\dfrac{y-2}{-1}=\dfrac{x}{1}\]
On cross multiplication, we get
\[y-2=-x\]
\[x+y-2=0........(i)\]
Similarly, the equation of side BC is,
\[\dfrac{y-0}{4-0}=\dfrac{x-2}{4-2}\]
\[\dfrac{y}{4}=\dfrac{x-2}{2}\]
\[\dfrac{y}{2}=\dfrac{x-2}{1}\]
On cross multiplication, we get
\[y=2x-4\]
\[2x-y-4=0........(ii)\]
And, the equation of side AC is,
\[\dfrac{y-2}{4-2}=\dfrac{x-0}{4-0}\]
\[\dfrac{y-2}{2}=\dfrac{x}{4}\]
\[\dfrac{y-2}{1}=\dfrac{x}{2}\]
On cross multiplication, we get
\[2y-4=x\]
\[x-2y+4=0........(iii)\]
Therefore the figure with equations is,
Two given points \[\left( {{x}_{1}},\text{ }{{y}_{1}} \right)\]and \[\left( {{x}_{2}},\text{ }{{y}_{2}} \right)\]will lie on the same side of the line \[ax+by+c=0\] if \[a{{x}_{1}}+b{{y}_{1}}+c\] and \[a{{x}_{2}}+b{{y}_{2}}+c\] will have same signs.
From the above figure it is clear that the points B and D lie on the same side of the line AC. So, it should satisfy the above condition, i.e.,
\[2-2(0)+4=6>0\]
So, when we substitute the value of point D in line AC, it should be greater than zero, i.e.,
\[\alpha -2(2\alpha )+4>0\]
\[\alpha -4\alpha +4>0\]
\[-3\alpha +4>0\]
\[4>3\alpha \]
\[\Rightarrow \alpha <\dfrac{4}{3}.........(iv)\]
Now from the above figure it is clear that the points C and D lie on the same side of the line AB. Substitute value of point C in equation of line AB, we get
\[4+4-2=6>0\]
So, when we substitute the value of point D in line AB, it should be greater than zero, i.e.,
\[\alpha +2\alpha -2>0\]
\[3\alpha -2>0\]
\[3\alpha >2\]
\[\Rightarrow \alpha >\dfrac{2}{3}.........(v)\]
So, from equation (iv) and (v), we get
\[\alpha \in \left( \dfrac{2}{3},\dfrac{4}{3} \right)\]
Hence the correct answer is option (c).
Note: We can solve this by finding boundaries of x and y. But the options are given in fraction form, using this method it gives the exact answer.
×
Sorry!, This page is not available for now to bookmark.
Like this
Liked
NCERT Book Solutions
Reference Book Solutions
Toll Free:
1800-120-456-456
Mobile:
+91 988-660-2456
Toll Free:
1800-120-456-456
Mobile:
+91 988-660-2456
(Mon-Sun: 9am - 11pm IST)
Questions?
Chat with us
