
The young’s modulus of a rubber string
A.
B.
C.
D.
Answer
501.3k+ views
2 likes
Hint: Concept of relation between young’s modulus and change in length is used.
Formula used:
Complete step by step solution:
Let us consider a rope or rubber band of length
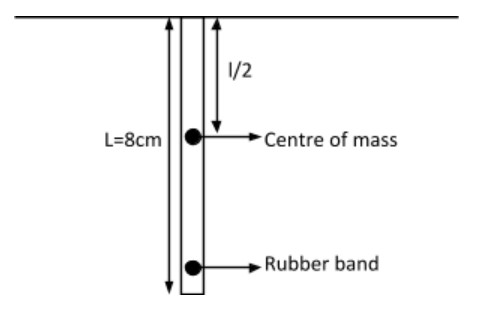
As the rope is of length,
We know that the relation between young’s modulus and change in length is given by
But here
So,
Now, density,
i.e.
Also, force,
Where
Put (II) and (III) in (I), we get
Given that,
Length of rubber band,
Density, of rubber band,
Young’s modulus,
The reaction for increase in length and young’s modulus is as follows,
Where
So, as
Hence, the correct option is (B).
Note: Before solving the question, we must consider the change in length of the rubber band due to its weight which will cause it to move away from the centre of mass. So, half-length is taken but in density, total length of rubber band is considered as density is due to mass and length of overall rubber band.
Formula used:
Complete step by step solution:
Let us consider a rope or rubber band of length

As the rope is of length,
We know that the relation between young’s modulus and change in length is given by
But here
So,
Now, density,
i.e.
Also, force,
Where
Put (II) and (III) in (I), we get
Given that,
Length of rubber band,
Density, of rubber band,
Young’s modulus,
The reaction for increase in length and young’s modulus is as follows,
Where
So, as
Hence, the correct option is (B).
Note: Before solving the question, we must consider the change in length of the rubber band due to its weight which will cause it to move away from the centre of mass. So, half-length is taken but in density, total length of rubber band is considered as density is due to mass and length of overall rubber band.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




