
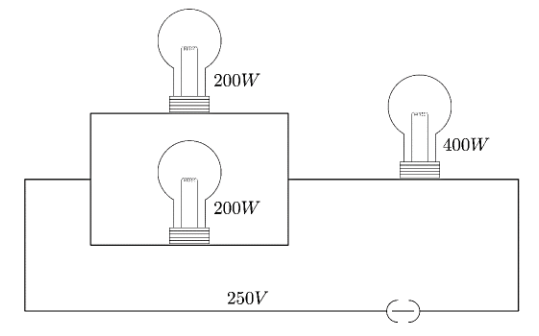
Three electric bulbs of
A.
B.
C.
D.

Answer
432k+ views
Hint: Power is the rate of change of work done per unit time. When a bulb is connected with the battery it dissipates the electrical energy into light energy and heat So when we calculate the power it means how much energy dissipated by the bulb per second.
Complete step by step answer:
In this combination three electric bulbs have power
Then,
When the resistor connected in series then we have a formula of equivalent resistance
Where,
Dividing both side by
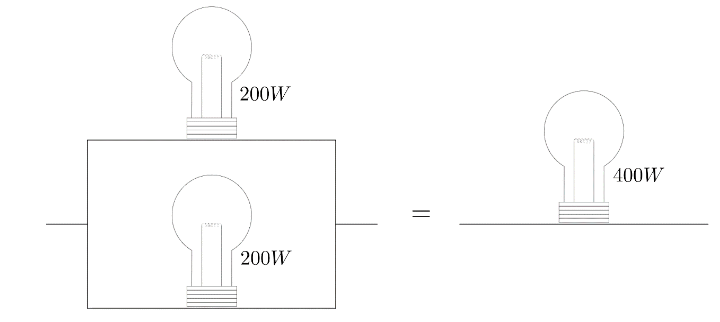
When the resistor connected in parallel then then we have a formula of equivalent resistance
Where,
After putting the value of
After multiplying both side by
In this problem power
But
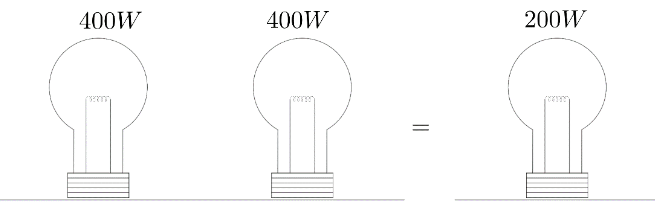
Hence, the resultant power of the combination is 200 W.
Hence, the correct answer is option C.
Note: Electric bulb converts the electrical energy into light energy and heat energy. In the series combination of resistor current is constant but in the case of parallel combination voltage is constant. Equivalent power means when we remove all three bulbs and connect a single bulb having the same resistance then power of a single bulb is equal to the equivalent power of three bulbs.
Complete step by step answer:
In this combination three electric bulbs have power
Then,
When the resistor connected in series then we have a formula of equivalent resistance
Where,
Dividing both side by
When the resistor connected in parallel then then we have a formula of equivalent resistance
Where,
After putting the value of
After multiplying both side by
In this problem power

But

Hence, the resultant power of the combination is 200 W.
Hence, the correct answer is option C.
Note: Electric bulb converts the electrical energy into light energy and heat energy. In the series combination of resistor current is constant but in the case of parallel combination voltage is constant. Equivalent power means when we remove all three bulbs and connect a single bulb having the same resistance then power of a single bulb is equal to the equivalent power of three bulbs.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




