Question

Three girls Reshma, Salma and Mandip are playing a game by standing on a circle of the radius $5m$ drawn in a park. Reshma throws a ball to Salma, Salma to Mandip, Mandip to Reshma. The distance between Reshma and Salma and between Salma and Mandip is $6m$ each, what is the distance between Reshma and Mandip?

Answer 1

Hint: Here we will first construct a diagram based on the given data, first we will draw a circle of radius $5m$ and then we will mark the places where Reshma, Salma and Mandip stood as $R,S,M$ on the circle. Now we will list all the lengths of the parameters we have in the circle. Now we will calculate the required distance by using the triangles that are formed by joining the points on the circle with each of them and with the center of the circle.

Complete step-by-step answer:
Given that,
Three girls Reshma, Salma and Mandip are playing a game by standing on a circle of the radius $5m$ drawn in a park. Reshma throws a ball to Salma, Salma to Mandip, Mandip to Reshma.
The diagrammatical representation of the above data is shown below

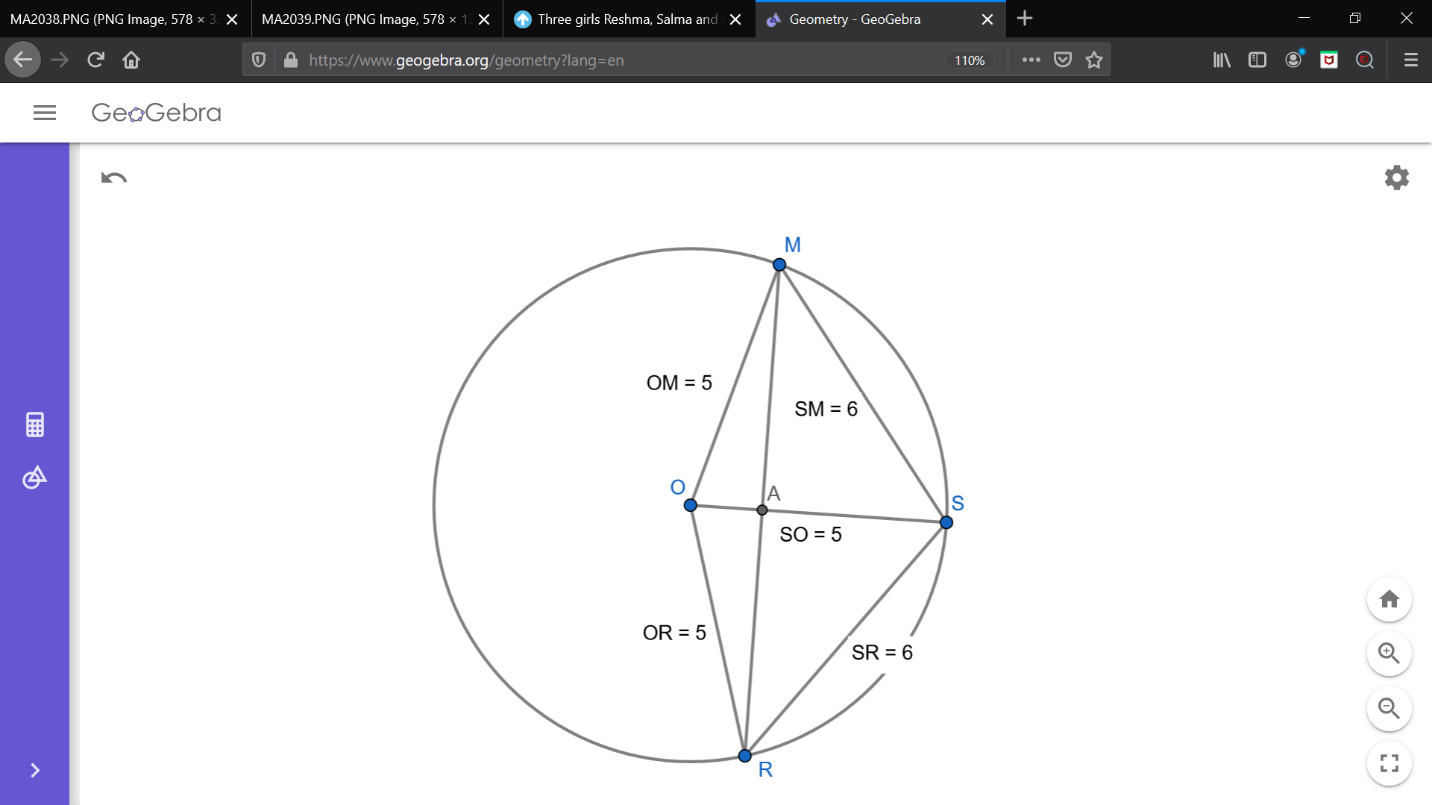
Here the points
$O$ is the center of the circle.
$S$ is the point where Salma stood on the circle.
$R$ is the point where Reshma stood on the circle.
$M$ is the point where Mandip stood on the circle.
Here the lengths
$SM$ is the distance between Salma and Mandip, according to problem $SM=6m$
$SR$ is the distance between Salma and Reshma, according to the problem $SR=6m$
$MR$ is the distance between Mandip and Reshma, we need to calculate this length.
We know that the distance between the center of the circle to the any point on the circle is called as the radius, hence
$OM=OR=OS=r=5m$
From the diagram we can observe that, $OS$ is the angular bisector of $\angle RSM$ and it is also passing through the center, so we know that it will perpendicularly bisects the chords of the circles i.e. $OS$ is the perpendicular bisector of the line $MR$ and it bisects the line $MR$ at point $A$, hence
$MA=RA...\left( \text{i} \right)$
From the right-angled triangle $SAR$, using Pythagoras theorem
$\begin{align}
  & S{{R}^{2}}=R{{A}^{2}}+S{{A}^{2}} \\
 &\Rightarrow R{{A}^{2}}=S{{R}^{2}}-S{{A}^{2}} \\
 &\Rightarrow R{{A}^{2}}={{6}^{2}}-S{{A}^{2}}...\left( \text{ii} \right) \\
\end{align}$
Now from the right-angled triangle $OAR$, using the Pythagoras theorem
$\begin{align}
  & O{{R}^{2}}=O{{A}^{2}}+A{{R}^{2}} \\
 &\Rightarrow {{5}^{2}}=O{{A}^{2}}+A{{R}^{2}} \\
 &\Rightarrow A{{R}^{2}}={{5}^{2}}-O{{A}^{2}} \\
\end{align}$
Now from the diagram we can write $OA=OS-SA$ in the above equation, then we will have
$\begin{align}
  & A{{R}^{2}}={{5}^{2}}-\left( O{{S}^{2}}-S{{A}^{2}} \right) \\
 & ={{5}^{2}}-{{\left( 5-SA \right)}^{2}}....\left( \text{iii} \right) \\
\end{align}$
Equating the equations $\left( \text{ii} \right)$ and $\left( \text{iii} \right)$, then we will get
${{6}^{2}}-S{{A}^{2}}={{5}^{2}}-{{\left( 5-SA \right)}^{2}}$
Using the formula ${{\left( a-b \right)}^{2}}={{a}^{2}}+{{b}^{2}}-2ab$ in the above equation, then we will get
$\begin{align}
  & 36-S{{A}^{2}}=25-\left( 25+S{{A}^{2}}-2.5.SA \right) \\
 &\Rightarrow 36-S{{A}^{2}}=25-25-S{{A}^{2}}+10SA \\
 &\Rightarrow 36-S{{A}^{2}}+S{{A}^{2}}=0+10SA \\
 &\Rightarrow 36+0=10SA \\
 &\Rightarrow 10SA=36 \\
 &\Rightarrow SA=\dfrac{36}{10} \\
 &\Rightarrow SA=3.6 \\
\end{align}$
Substituting the value of $SA=3.6$ in equation $\left( \text{ii} \right)$, then we will get
$\begin{align}
  & R{{A}^{2}}={{6}^{2}}-{{3.6}^{2}} \\
 &\Rightarrow R{{A}^{2}}=36-12.96 \\
 &\Rightarrow R{{A}^{2}}=23.04 \\
 &\Rightarrow RA=\sqrt{23.04} \\
 &\Rightarrow RA\simeq 4.8m \\
\end{align}$
Now from the equation $\left( \text{i} \right)$, we can write that
$\begin{align}
  &\Rightarrow RM=2RA \\
 &\Rightarrow RM=2\times 4.8 \\
 & =9.6m \\
\end{align}$
Hence the distance between Reshma and Mandip is $9.6m$

Note: For this problem diagrammatic representation takes a major part. After plotting the given data into picture, we can use any of the triangles in order to get the solution. Students must mark the given distances properly in the figure. By mistake, if they mark OR = OM = 6 instead of 5, then the results will vary. We are supposed to find the distance between Reshma and Mandip, so we must find the total length RM by adding RA and AM.