
Three of six vertices of a regular hexagon are chosen at random. The probability that the triangle with three vertices is equilateral, is equal to
Answer
514.8k+ views
1 likes
Hint:First of all, find the total number of triangles that is possible by taking 3 points of a regular hexagon which has 6 vertices. Total number of triangles is
Complete step-by-step answer:
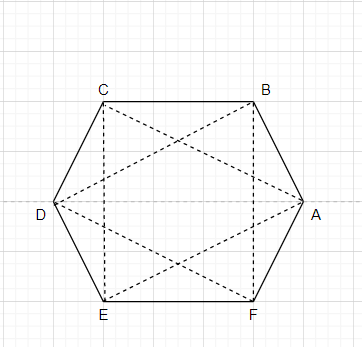
We have connected the vertex A,E and C and can see that we got an equilateral triangle.
Similarly, We have connected the vertex D,F and B and can see that we got an equilateral triangle.
Suppose if we connect the vertex A,D and E, we don’t get an equilateral triangle. Because, according to the diagram we can see that all three sides are not equal to each other.
We have only two equilateral triangles to be formed using a regular hexagon. Out of six points in a hexagon, we have to select only three points at a time.
The total number of triangles to be formed using a regular hexagon=
Out of 20 triangles, there are only two equilateral triangles that are
Probability of choosing equilateral triangle
Note: In this question, one can make mistakes in taking the number of equilateral triangles. One can think that there can be six equilateral triangles that are
Complete step-by-step answer:

We have connected the vertex A,E and C and can see that we got an equilateral triangle.
Similarly, We have connected the vertex D,F and B and can see that we got an equilateral triangle.
Suppose if we connect the vertex A,D and E, we don’t get an equilateral triangle. Because, according to the diagram we can see that all three sides are not equal to each other.
We have only two equilateral triangles to be formed using a regular hexagon. Out of six points in a hexagon, we have to select only three points at a time.
The total number of triangles to be formed using a regular hexagon=
Out of 20 triangles, there are only two equilateral triangles that are
Probability of choosing equilateral triangle
Note: In this question, one can make mistakes in taking the number of equilateral triangles. One can think that there can be six equilateral triangles that are
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




