Question

Three of the vertices of a parallelogram have coordinates ( 5, -2 ), (6,2) and (0,2). Plot these points on the graph paper and find the coordinate of the fourth vertex.

Answer 1

Hint: To find the coordinates of fourth vertex, we will use the formula of midpoint use for finding midpoint of any line joined by two end points $A\left( {{x}_{1}},{{x}_{2}} \right)$ and $B\left( {{y}_{1}},{{y}_{2}} \right)$, then mid point is $\left( \dfrac{{{x}_{1}}+{{x}_{2}}}{2},\dfrac{{{y}_{1}}+{{y}_{2}}}{2} \right)$. After finding the midpoint of one diagonal, then using the property of parallelogram, two diagonals of parallelogram bisects each other, which concludes that mid-point of both diagonals are the same, and we will evaluate coordinates of the fourth vertex.

Complete step-by-step answer:

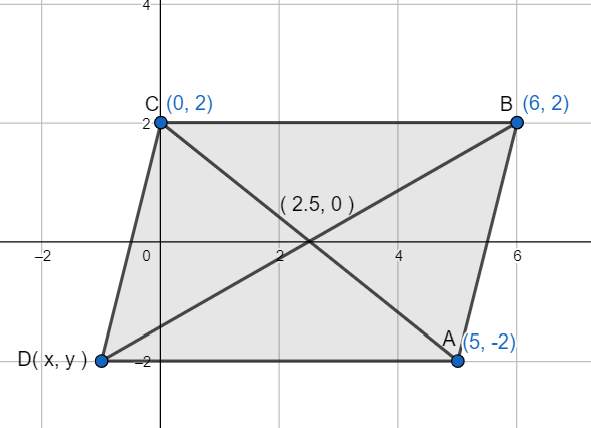
Now, we have to find the value of the fourth vertex. So, let the fourth vertex be ( x, y ) .
Now, we know that in parallelogram, opposite sides are parallel and are of equal lengths.
So, we can say that line joining vertices ( 5, -2 ) and ( 6, 2 ) and line joining vertices ( x, y ) and ( 0, 2 ) will be parallel, that is line AB and DC will be parallel and equal to each other in length.
Now, note that in parallelogram, the two diagonals of parallelogram bisects each other, which concludes that mid-point of both diagonals are the same.
So, for parallelogram ABCD, diagonals will be AC and line BD and both diagonals AC and BD will bisect at one point which will be mid point of both diagonals AC and BD.
Now, from the figure, let us consider diagonal joining points A ( 5, -2 ) and C ( 0, 2 ).
So, midpoint of diagonal AC will be $\left( \dfrac{5+0}{2},\dfrac{-2+2}{2} \right)$ as we know that midpoint of line joining points $A\left( {{x}_{1}},{{x}_{2}} \right)$ and $B\left( {{y}_{1}},{{y}_{2}} \right)$ is evaluate by mid point formula which is $\left( \dfrac{{{x}_{1}}+{{x}_{2}}}{2},\dfrac{{{y}_{1}}+{{y}_{2}}}{2} \right)$.
So, on solving
midpoint of line AC will be (2.5, 0)
now, as we discussed that mid point of both diagonal will be same, so we can write as
$2.5=\dfrac{x+6}{2}$ and $0=\dfrac{y+2}{2}$, where ( x, y ) are coordinates of fourth vertex, D.
So, on simplifying, we get
5 = x + 6 and y + 2 = 0
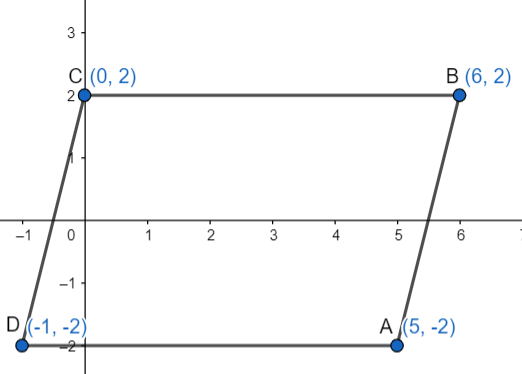
So, coordinate of fourth vertex is ( -1, -2 )

Note: Always remember that in parallelogram, two diagonals of parallelogram bisects each other, which concludes that mid-point of both diagonals are the same. Also, keep in mind that midpoint of line joining points $A\left( {{x}_{1}},{{x}_{2}} \right)$ and $B\left( {{y}_{1}},{{y}_{2}} \right)$ is evaluate by mid point formula which is $\left( \dfrac{{{x}_{1}}+{{x}_{2}}}{2},\dfrac{{{y}_{1}}+{{y}_{2}}}{2} \right)$. While solving, for midpoint, calculate the midpoint carefully as this mid-point will get in use for finding the fourth vertex. Try to avoid calculation error as it will change the error and will lead to wrong plotting of the graph.