
To derive the expressions for Maximum height, time of ascent, time of descent, time of flight in the case of vertical projection:
Answer
440.8k+ views
2 likes
Hint: The expressions for the maximum height, time of ascent, time of descent, time of flight in the case of vertical projection can be easily determined by using a diagram of the projectile. In this case, we will make use of the vertical component of the velocity, as we will be deriving the expressions for the above-mentioned parameters belonging to a vertical projection.
Complete step by step answer:
Let a projectile be projected at an angle
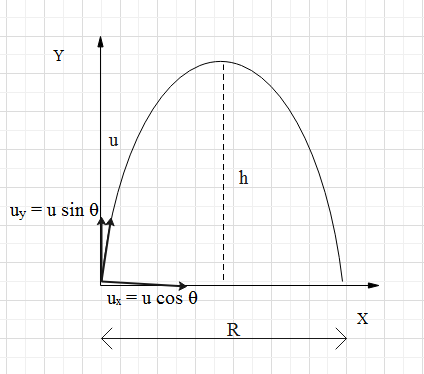
From the diagram, we get,
Substitute the values in the above equation.
Maximum height
The height reached by the body when projected vertically upwards where the vertical velocity is zero.
Using the law of motion equation we will further continue to find the expression of maximum height.
Further solve the above equation.
Hence the expression for the maximum height.
The time of ascent
The time taken by the body to reach the maximum height when projected vertically upwards.
Let the time taken by the projectile to reach the maximum height = t
At the maximum height, the velocity will be zero, v = 0
Using the law of motion equation we will further continue to find the expression of time of ascent.
As the value of the velocity is zero, substitute the same in the above equation.
Hence the expression for the time of ascent.
The time of descent
The time taken by the body to reach the ground after attaining the maximum height when projected vertically upwards.
Substitute the values of the variables T and t in the above equation to find the expression of the time of descent.
So, we get,
Hence the expression for the time of descent.
Therefore, we can notice that the time of ascent and the time of descent are the same for a vertically projected object.
The time of flight
The time taken by the body to get projected and land.
Again using the law of motion formulae we will find the expression for the time of flight.
Let the time taken to complete the trajectory be T
We have the formulae,
Now substitute the values in the above equation.
Rearrange the terms to find the expression in terms of T.
Hence the expression for the time of flight.
Therefore, the expressions for value of the Maximum height, time of ascent, time of descent, time of flight in the case of vertical projection are
Note:
The things to be on your finger-tips for further information on solving these types of problems are: The vertical and the horizontal components of the projectile are different, as they are ruled by the different forms of the forces.
Complete step by step answer:
Let a projectile be projected at an angle

From the diagram, we get,
Substitute the values in the above equation.
Maximum height
The height reached by the body when projected vertically upwards where the vertical velocity is zero.
Using the law of motion equation we will further continue to find the expression of maximum height.
Further solve the above equation.
Hence the expression for the maximum height.
The time of ascent
The time taken by the body to reach the maximum height when projected vertically upwards.
Let the time taken by the projectile to reach the maximum height = t
At the maximum height, the velocity will be zero, v = 0
Using the law of motion equation we will further continue to find the expression of time of ascent.
As the value of the velocity is zero, substitute the same in the above equation.
Hence the expression for the time of ascent.
The time of descent
The time taken by the body to reach the ground after attaining the maximum height when projected vertically upwards.
Substitute the values of the variables T and t in the above equation to find the expression of the time of descent.
So, we get,
Hence the expression for the time of descent.
Therefore, we can notice that the time of ascent and the time of descent are the same for a vertically projected object.
The time of flight
The time taken by the body to get projected and land.
Again using the law of motion formulae we will find the expression for the time of flight.
Let the time taken to complete the trajectory be T
We have the formulae,
Now substitute the values in the above equation.
Rearrange the terms to find the expression in terms of T.
Hence the expression for the time of flight.
Therefore, the expressions for value of the Maximum height, time of ascent, time of descent, time of flight in the case of vertical projection are
Note:
The things to be on your finger-tips for further information on solving these types of problems are: The vertical and the horizontal components of the projectile are different, as they are ruled by the different forms of the forces.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




