Question

Two adjacent angles on a straight line are in the ratio $5:4$ . Find the measure of the smallest angle.

Answer 1

Hint: We can draw a diagram representing the angles given in the question. We can multiply the ratio with a variable x. Then we can take their sum and equate it to $180^\circ $ . Then we can solve for x and by substituting it back in the ratio, we can obtain the measures of the angles. Then the angle with smaller measure will be the required angle.

Complete step-by-step answer:
We can draw a figure showing two adjacent angles on a straight line.

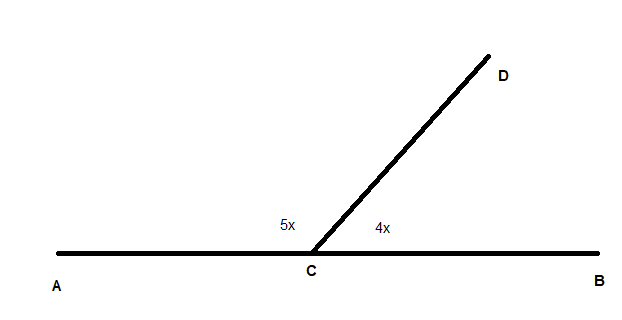
We are given that the two adjacent angles on a straight line are in the ratio $5:4$ . We can multiply the ratio with the same variable.
Then the angles are given by, 5x and 4x.
It is given that these angles are adjacent angles on a straight line. So, they form a linear pair.
We know that linear pair are supplementary and have sum equal to $180^\circ $
 $ \Rightarrow 5x + 4x = 180^\circ $
 $ \Rightarrow 9x = 180^\circ $
 $ \Rightarrow x = \dfrac{{180^\circ }}{9}$
 $ \Rightarrow x = 20^\circ $
Now we have x as $20^\circ $
Then the angles are,
 $ \Rightarrow 5x = 5 \times 20^\circ = 100^\circ $
 $ \Rightarrow 4x = 4 \times 20^\circ = 80^\circ $
We need to find the measure of the smallest angle. So out of the two angles, the measure of the smallest angle is $80^\circ $
Therefore, the required measure of angle is $80^\circ $ .
Note: We used the property of ratio that the ratio remains unchanged when multiplied or divided by the same number. We must use the same variable for multiplying both parts of the ratio. Linear pairs of angles are the angles formed when one line is intersected with a ray starting from the line. Without calculating the values of each angle, we can say that the angle having the lowest coefficient in the ratio is the angle with the least measure.