
Two lines that intersect each other at right angles are called \[\]
A. Parallel \[\]
B. Perpendicular \[\]
C. Equal \[\]
D. None of these \[\]
Answer
470.7k+ views
Hint: We recall the definition of right angle, intersecting lines, and the angle they subtended at the point of intersection, parallel lines, and angle between them, and whether equality can be established between lines. We use the definition that these lines are perpendicular if they divide the straight angle subtended at the other line equally.
Complete step-by-step solution
We know a straight line is a one-dimensional geometrical shape with no curvature extended in two directions as a collection of points. A line is denoted with two points $A, B$ on the line as $\overleftrightarrow{AB}$, or by a single letter $l.$ We know that a line subtends a straight angle which is defined as an angle with a measure of ${{180}^{\circ }}$at any point $O.$ So we have $\angle AOB={{180}^{\circ }}$ \[\]
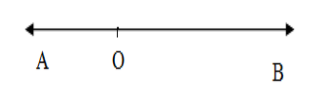
We know that two lines can at most intersect at one point or do not intersect at all. When they intersect they are called intersecting lines and if they do not they are called parallel lines. The angle between parallel lines is ${{0}^{\circ }}$or ${{180}^{\circ }}$. So option A is not correct. \[\]
Let us have two lines $\overleftrightarrow{AB}$ and $\overleftrightarrow{CD}$ intersect each other at the point $O$.We have the figure as \[\]
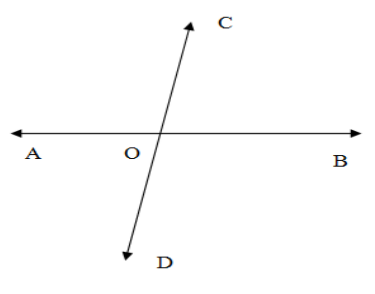
The lines $\overleftrightarrow{AB}, ,\overleftrightarrow{CD}$ are perpendicular if they divide the straight angle subtended at the other line equally. $\overleftrightarrow{CD}$ divides the straight angle $\angle AOB$ into angles $\angle AOC,\angle BOC$ above itself and into angles $\angle AOD,\angle BOD$ below itself. If they intersect each other at right angle as given in the question which means they at intersect with angle with measurement ${{90}^{\circ }}$, then we have,
\[\begin{align}
& \angle AOC=\angle BOC={{90}^{\circ }} \\
& \angle AOD=\angle BOD={{90}^{\circ }} \\
\end{align}\]
Let us add then and get
\[\begin{align}
& \angle AOC+\angle BOC={{90}^{\circ }}+{{90}^{\circ }}=\angle AOB \\
& \angle AOD+\angle BOD={{90}^{\circ }}+{{90}^{\circ }}=\angle AOB \\
\end{align}\]
So the $\overleftrightarrow{AB}, \overleftrightarrow{CD}$ divide the straight angle at the other line into two congruent right angles, and hence they are perpendicular. So option B is correct.\[\]

We know that lines cannot be measured but segments of the line can be measured. So option C is incorrect.\[\]
Note: We note that when two lines intersect they subtend pairs of two equal angles at the opposite of a line are called vertically opposite angles. Here the vertically opposite angles are $\angle AOC,\angle BOD$ and $\angle BOC,\angle AOD.$ The angels who are on the same side are called linear pairs. Here the linear pairs at the same side of $\overleftrightarrow{AB}$ are $\left( \angle AOC,\angle BOC \right)$ and $\left( \angle AOD,\angle BOD \right)$ . The linear pairs at the same side of $\overleftrightarrow{CD}$ are $\left( \angle BOC,\angle BOD \right)$ and $\left( \angle AOC,\angle AOD \right)$ . The examples of perpendicular lines are coordinate axes in-plane, sides of rectangle, diameter, and tangent of the circle, etc.
Complete step-by-step solution
We know a straight line is a one-dimensional geometrical shape with no curvature extended in two directions as a collection of points. A line is denoted with two points $A, B$ on the line as $\overleftrightarrow{AB}$, or by a single letter $l.$ We know that a line subtends a straight angle which is defined as an angle with a measure of ${{180}^{\circ }}$at any point $O.$ So we have $\angle AOB={{180}^{\circ }}$ \[\]
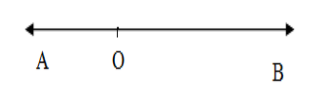
We know that two lines can at most intersect at one point or do not intersect at all. When they intersect they are called intersecting lines and if they do not they are called parallel lines. The angle between parallel lines is ${{0}^{\circ }}$or ${{180}^{\circ }}$. So option A is not correct. \[\]
Let us have two lines $\overleftrightarrow{AB}$ and $\overleftrightarrow{CD}$ intersect each other at the point $O$.We have the figure as \[\]

The lines $\overleftrightarrow{AB}, ,\overleftrightarrow{CD}$ are perpendicular if they divide the straight angle subtended at the other line equally. $\overleftrightarrow{CD}$ divides the straight angle $\angle AOB$ into angles $\angle AOC,\angle BOC$ above itself and into angles $\angle AOD,\angle BOD$ below itself. If they intersect each other at right angle as given in the question which means they at intersect with angle with measurement ${{90}^{\circ }}$, then we have,
\[\begin{align}
& \angle AOC=\angle BOC={{90}^{\circ }} \\
& \angle AOD=\angle BOD={{90}^{\circ }} \\
\end{align}\]
Let us add then and get
\[\begin{align}
& \angle AOC+\angle BOC={{90}^{\circ }}+{{90}^{\circ }}=\angle AOB \\
& \angle AOD+\angle BOD={{90}^{\circ }}+{{90}^{\circ }}=\angle AOB \\
\end{align}\]
So the $\overleftrightarrow{AB}, \overleftrightarrow{CD}$ divide the straight angle at the other line into two congruent right angles, and hence they are perpendicular. So option B is correct.\[\]

We know that lines cannot be measured but segments of the line can be measured. So option C is incorrect.\[\]
Note: We note that when two lines intersect they subtend pairs of two equal angles at the opposite of a line are called vertically opposite angles. Here the vertically opposite angles are $\angle AOC,\angle BOD$ and $\angle BOC,\angle AOD.$ The angels who are on the same side are called linear pairs. Here the linear pairs at the same side of $\overleftrightarrow{AB}$ are $\left( \angle AOC,\angle BOC \right)$ and $\left( \angle AOD,\angle BOD \right)$ . The linear pairs at the same side of $\overleftrightarrow{CD}$ are $\left( \angle BOC,\angle BOD \right)$ and $\left( \angle AOC,\angle AOD \right)$ . The examples of perpendicular lines are coordinate axes in-plane, sides of rectangle, diameter, and tangent of the circle, etc.
Recently Updated Pages
Can anyone list 10 advantages and disadvantages of friction

What are the Components of Financial System?

How do you arrange NH4 + BF3 H2O C2H2 in increasing class 11 chemistry CBSE

Is H mCT and q mCT the same thing If so which is more class 11 chemistry CBSE

What are the possible quantum number for the last outermost class 11 chemistry CBSE

Is C2 paramagnetic or diamagnetic class 11 chemistry CBSE

Trending doubts
The correct order of melting point of 14th group elements class 11 chemistry CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

What is the specific heat capacity of ice water and class 11 physics CBSE

State the laws of reflection of light

Proton was discovered by A Thomson B Rutherford C Chadwick class 11 chemistry CBSE

Why does niobium have a d4s1 electron configuration class 11 chemistry CBSE




