
Two rods of same length and material transfer a given amount of heat in
A.
B.
C.
D.
Answer
442.8k+ views
Hint: Use the equation for heat flow through a material between its two ends.According to heat definition, it is one of the essential forms of energy for the survival of life on earth. Transfer of heat takes place from one body to another due to difference in temperature as per thermodynamics.
Formula used:
The equation for heat flow through a material between its two ends is given by,
where ,
Complete step by step answer:
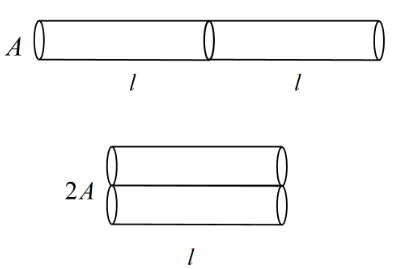
Now, here we have two rods of same length ( say,
Now, they are joined in series and the same heat
On simplifying we get,
Hence, it will take
Hence, the correct answer is option (D).
Note: The heat flow through a material is dependent on the temperature differences of the opposite surfaces. Hence, the temperature differences have been kept constant for both the conditions here. If the material was different with given coefficient
Formula used:
The equation for heat flow through a material between its two ends is given by,
where ,
Complete step by step answer:

Now, here we have two rods of same length ( say,
Now, they are joined in series and the same heat
On simplifying we get,
Hence, it will take
Hence, the correct answer is option (D).
Note: The heat flow through a material is dependent on the temperature differences of the opposite surfaces. Hence, the temperature differences have been kept constant for both the conditions here. If the material was different with given coefficient
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




