
Two ships are approaching a light house from opposite directions. The angles of depression of two ships from top of the light house are
Answer
480.6k+ views
Hint: Here we will use the trigonometric functions to find the height of the light house. Firstly we will assume the length of a boat from the bottom of the light house. Then by using the trigonometric function we will find the relation between the height of the light house and the distance between the base of the light house and the boat.
Complete step-by-step answer:
Let
It is given that the distance between two ships is 100m. Therefore the distance between the ship making an angle of
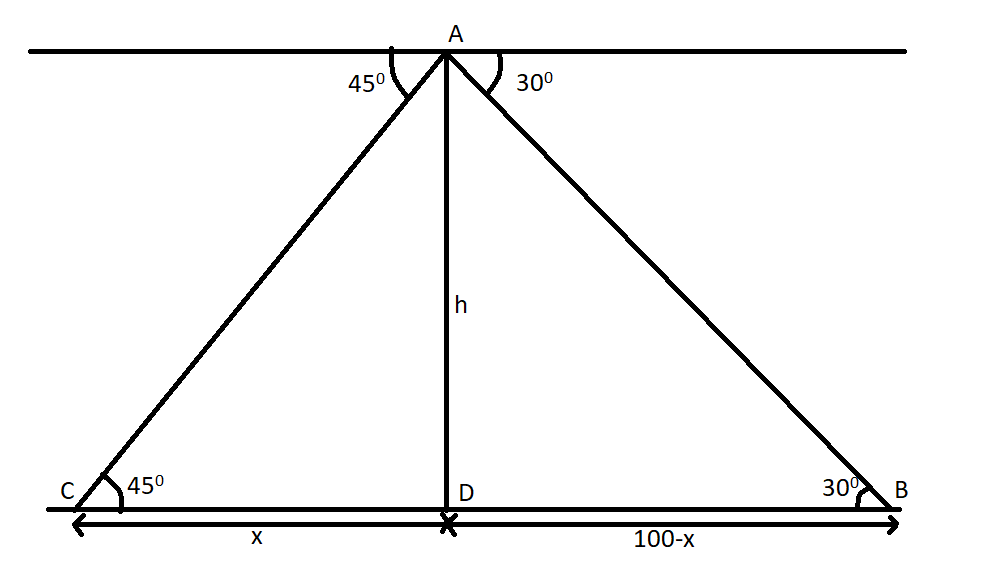
In
We know that
Now we will take the triangle
We know that
Now, substituting the value of
Hence, the height of the light house is equal to
Note: Here we should note that in these types of questions, where the height of some tower or poles is asked to find, then we have to use the trigonometric function to find its value. Also any building or tower constructed on the ground is generally perpendicular to the ground this is why we can use the trigonometric function to find the value of its height.
Complete step-by-step answer:
Let
It is given that the distance between two ships is 100m. Therefore the distance between the ship making an angle of

In
We know that
Now we will take the triangle
We know that
Now, substituting the value of
Hence, the height of the light house is equal to
Note: Here we should note that in these types of questions, where the height of some tower or poles is asked to find, then we have to use the trigonometric function to find its value. Also any building or tower constructed on the ground is generally perpendicular to the ground this is why we can use the trigonometric function to find the value of its height.
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

What are the public facilities provided by the government? Also explain each facility




