
Two vectors of equal magnitude have a resultant equal to either of them in magnitude. The angle between them is:
A. 60°
B. 90°
C. 105°
D. 120°
Answer
488.4k+ views
13 likes
Hint: It is given that two vectors are equal in magnitude i.e. if A and B are two vectors then
Formula used:
Complete answer:
Let the two vectors be
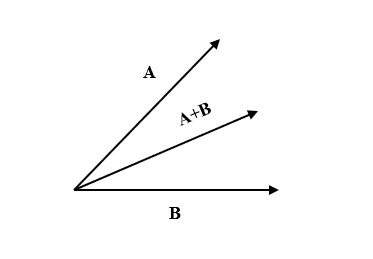
Both the vectors have the same magnitude.
Let the resultant have magnitude equal to vector A.
Thus, the resultant is given by,
The magnitude of resultant of two vectors is given by,
From the equation. (2) and equation. (3) we get,
Squaring both the sides we get,
Substituting equation. (1) in above equation we get,
Hence, the angle between the two vectors is 120°.
So, the correct answer is “Option D”.
Note:
Students must remember that while adding two vectors don’t only consider the magnitude of the vectors but also consider the direction of both the vectors. If you don’t consider the direction then there might be an error in your calculation. If we double the resultant and reverse one of the vectors then the resultant gets doubled again.
Formula used:
Complete answer:
Let the two vectors be

Both the vectors have the same magnitude.
Let the resultant have magnitude equal to vector A.
Thus, the resultant is given by,
The magnitude of resultant of two vectors is given by,
From the equation. (2) and equation. (3) we get,
Squaring both the sides we get,
Substituting equation. (1) in above equation we get,
Hence, the angle between the two vectors is 120°.
So, the correct answer is “Option D”.
Note:
Students must remember that while adding two vectors don’t only consider the magnitude of the vectors but also consider the direction of both the vectors. If you don’t consider the direction then there might be an error in your calculation. If we double the resultant and reverse one of the vectors then the resultant gets doubled again.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




