
Using ruler and compasses only, construct a triangle POR such that
A.Circumcentre
B.Incentre
C.Mid point
D.Data insufficient
Answer
479.1k+ views
Hint: Using the knowledge of construction by ruler and scale to draw the required triangle and then find out the point which is equidistant from all the sides of the triangle by drawing angle bisectors of all the angles. Taking this point as the centre, draw a circle to find out what is the name of that point.
Complete step-by-step answer:
Construction of the triangle POR –
I.Draw a line PR of length 6 cm with the help of scale.
II.Make an angle of
III.Cut a line segment PO=5 cm.
IV.Join the points O and R, such that OR=9.6 cm
This way we have constructed the required triangle POR.
V.To find out the point equidistant from the three sides, we draw angle bisectors of all the angles of the triangle as shown in the figure.
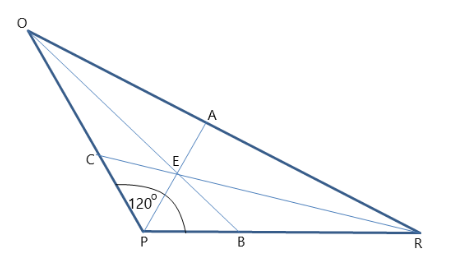
V.Now, taking this point as the centre, we make a circle touching the three sides of the triangle.
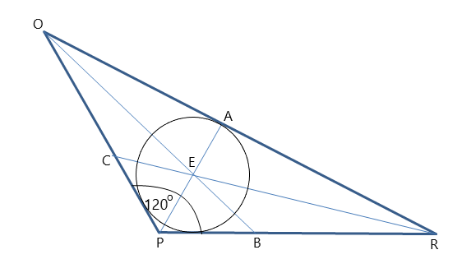
The circle inside a triangle is called incircle and the centre of this circle is called incentre.
Thus point E is called the incentre.
So, the correct answer is “Option B”.
Note: Drawing shapes, angles or lines accurately is known as construction. These constructions use only compass, ruler and a pencil. No numbers are involved in construction that’s why it is called the true form of geometric construction. The basics of construction include drawing line segments, right angles and angle bisectors.
Complete step-by-step answer:
Construction of the triangle POR –
I.Draw a line PR of length 6 cm with the help of scale.
II.Make an angle of
III.Cut a line segment PO=5 cm.
IV.Join the points O and R, such that OR=9.6 cm
This way we have constructed the required triangle POR.
V.To find out the point equidistant from the three sides, we draw angle bisectors of all the angles of the triangle as shown in the figure.

V.Now, taking this point as the centre, we make a circle touching the three sides of the triangle.

The circle inside a triangle is called incircle and the centre of this circle is called incentre.
Thus point E is called the incentre.
So, the correct answer is “Option B”.
Note: Drawing shapes, angles or lines accurately is known as construction. These constructions use only compass, ruler and a pencil. No numbers are involved in construction that’s why it is called the true form of geometric construction. The basics of construction include drawing line segments, right angles and angle bisectors.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

The LCM and HCF of two rational numbers are equal Then class 8 maths CBSE





