
What is the volume of a cube whose side length is 6 cm?
Answer
478.5k+ views
2 likes
Hint: Here, we have to use the concept and formula of volume for cube. Volume is the amount of space occupied by an object in three-dimensional space. So, by simply using the formula of the volume we will be able to find out the volume of the cube with side length 6 cm.
Formula used:
We will use the formula of the volume of the cube
Complete step-by-step answer:
A Cube is the three dimensional object with six flat surfaces which has length of all the edges or sides equal to each other.
It is given that the side of the cube is 6 cm which means all the edges of the cube are of 6 cm length.
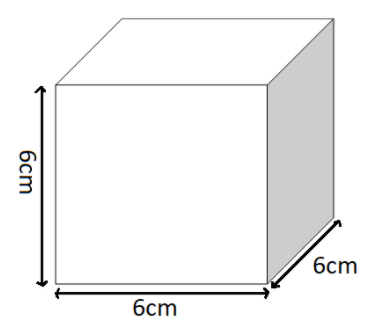
We know that volume of the cube is equal to space acquired by the cube in the three-dimensional space which is equal to the
Therefore, Volume of the cube
It is important to write the unit i.e.
Hence, the volume of the cube with side length 6 cm is
Note: Surface area of an object or shape is the sum of all the area of the faces of an object or shape and surface area is generally measured in square units. Volume is generally measured in cubic units.
A Cube is the shape with six flat surfaces, eight vertices or corners and twelve edges. Length of all these edges is equal to each other. Angles made by the two consecutive sides and the edges or sides are
Surface area of the cube
Volume of the cube
It is compulsory to write the units after the calculated values while solving a problem, it plays an important role.
Formula used:
We will use the formula of the volume of the cube
Complete step-by-step answer:
A Cube is the three dimensional object with six flat surfaces which has length of all the edges or sides equal to each other.
It is given that the side of the cube is 6 cm which means all the edges of the cube are of 6 cm length.

We know that volume of the cube is equal to space acquired by the cube in the three-dimensional space which is equal to the
Therefore, Volume of the cube
It is important to write the unit i.e.
Hence, the volume of the cube with side length 6 cm is
Note: Surface area of an object or shape is the sum of all the area of the faces of an object or shape and surface area is generally measured in square units. Volume is generally measured in cubic units.
A Cube is the shape with six flat surfaces, eight vertices or corners and twelve edges. Length of all these edges is equal to each other. Angles made by the two consecutive sides and the edges or sides are
Surface area of the cube
Volume of the cube
It is compulsory to write the units after the calculated values while solving a problem, it plays an important role.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE

How many crores make 10 million class 7 maths CBSE

Write a pair of integers whose sum gives i Zero ii class 7 maths CBSE

List of coprime numbers from 1 to 100 class 7 maths CBSE





