Question

Volume-Temperature graph at atmospheric pressure for a monoatomic gas ( V in m$^3$, T in $^\circ$C) is_______

(A).

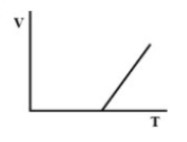
(B).

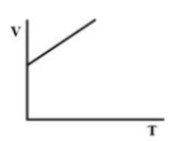
(C).

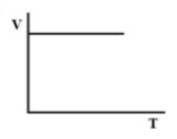
(D).

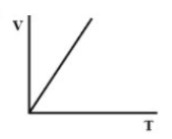
Answer 1

Hint: We all know that a gas follows the ideal gas equation at low pressure and high temperature. One of the parameters can be varied concerning the other parameters by keeping all extra parameters as constant. We can then analyze the variation of pressure, temperature, and volume to each other.

Complete step by step solution:
We know about the ideal gas equation that,
$PV = nRT$
Here, P is the absolute pressure of the gas, V is the volume occupied by the gas, R is the universal gas constant, and T is the absolute temperature, n is the number of moles of a gas.
For deriving this equation, the gas is assumed to be at low pressure and high temperature. Suppose if the pressure of the gas is held constant, then the relationship becomes linear between volume and temperature as R and n along with P is steady now. We get the relation as,
$V = \dfrac{{nR}}{P}T$
Since $\dfrac{{nR}}{P} = C$ it is constant, so the above relation becomes,
$V = CT$
Here we can express the relation as,
$V \propto T$
Here, V is having a linear relation with T. So, there is a straight-line relation between V and T.

Therefore, there must be a straight-line relation between V and T, and the correct option is (D).

Note: Also, we can analyze that when the volume is held constant, the pressure can be varied to temperature and follows a linear relation with temperature. Here the pressure that we are using is absolute and not gauge pressure.