
Water in the canal, 6m wide and 1.5m deep, is flowing with the speed of 10km/hr. How much area will it irrigate in 30 minutes, if 8 cm of standing water is needed.
Answer
500.1k+ views
Hint: First, before proceeding for this, we must know the following question is related to the figure cuboid as the canal is in the shape of a cuboid with breadth 6m and height 1.5m drawn. Then, to calculate the length of the canal in 1 minute, we need to use the conversion as
Complete step by step answer:
In this question, we are supposed to find the area the canal irrigates in 30 minutes, if 8 cm of standing water is needed with given conditions that the canal is 6m wide and 1.5m deep and water is flowing with the speed of 10km/hr.
So, before proceeding for this, we must know the following question is related to the figure cuboid as canal is in the shape of cuboid with breadth 6m and height 1.5m drawn as:
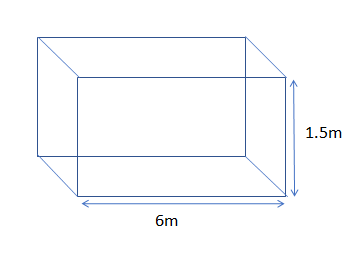
Now, the water is flowing in the canal on the length side of the canal with the speed of 10km/hr.
So, we get the length of the canal in 1 hour as 10km.
Also, we can say that the length of canal in 60 minutes is 10km.
Then, to calculate the length of canal in 1 minute, we need to use the conversion as:
Then, by using the above conversion, we get the length of canal in 1 minute as:
Now, in the question, we are asked to find t for 30 minutes, we get:
Then, we can easily calculate the volume V of the canal which is a cuboid by using the formula as:
Then, by substituting the value of length as 5000m, breadth as 6m and height as 1.5m, we get:
Then, we are given the question that calculated volume is equal to the area of the irrigation A which is actually the area irrigated into height.
So, by using this condition, we get the following expression as:
Then, by solving the above expression by substituting the value of height of water standing as 8cm which in metre is
Now, to convert the area of the irrigation on hectares, we have the conversion as:
Then, by using the above conversion, we get:
Hence, we get the area of the irrigation as 56.25 hectares.
Note:
Now, to solve these types of questions we need to know some of the basic conversions beforehand so that we can easily proceed in these types of questions. Then, some of the basic conversions are:
Complete step by step answer:
In this question, we are supposed to find the area the canal irrigates in 30 minutes, if 8 cm of standing water is needed with given conditions that the canal is 6m wide and 1.5m deep and water is flowing with the speed of 10km/hr.
So, before proceeding for this, we must know the following question is related to the figure cuboid as canal is in the shape of cuboid with breadth 6m and height 1.5m drawn as:

Now, the water is flowing in the canal on the length side of the canal with the speed of 10km/hr.
So, we get the length of the canal in 1 hour as 10km.
Also, we can say that the length of canal in 60 minutes is 10km.
Then, to calculate the length of canal in 1 minute, we need to use the conversion as:
Then, by using the above conversion, we get the length of canal in 1 minute as:
Now, in the question, we are asked to find t for 30 minutes, we get:
Then, we can easily calculate the volume V of the canal which is a cuboid by using the formula as:
Then, by substituting the value of length as 5000m, breadth as 6m and height as 1.5m, we get:
Then, we are given the question that calculated volume is equal to the area of the irrigation A which is actually the area irrigated into height.
So, by using this condition, we get the following expression as:
Then, by solving the above expression by substituting the value of height of water standing as 8cm which in metre is
Now, to convert the area of the irrigation on hectares, we have the conversion as:
Then, by using the above conversion, we get:
Hence, we get the area of the irrigation as 56.25 hectares.
Note:
Now, to solve these types of questions we need to know some of the basic conversions beforehand so that we can easily proceed in these types of questions. Then, some of the basic conversions are:
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




