
Water is leaking from a conical funnel at the rate of
Answer
504k+ views
Hint: In this question remember the method of differentiation also remember to use ratio of radius and height to put in the formula of conical flask i.e.
Complete step-by-step solution:
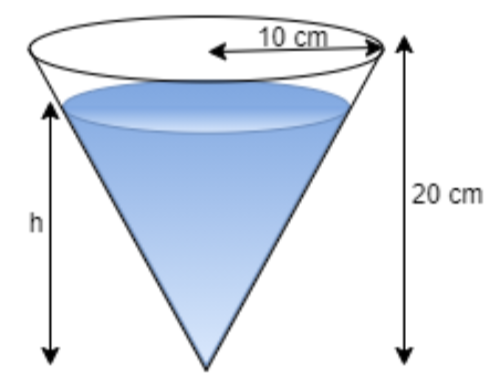
According to the given information we have a conical funnel leaking water at the rate of
The radius of conical funnel is 10 cm i.e. r = 10 cm and altitude is 20 cm i.e. h = 20 cm
We know that the volume of the conical flask is given by
Let
As we know that the ratio of radius and height of conical flask will remain same when the flask is full of water
So,
Substituting the given values in the equation 1 we get
Differentiating the above equation both side with respect to t we get
Substituting the values in the above equation we get
Therefore the rate of water level dropping in conical flask when water starts leaking is
Note: In the above solution we used the method of differentiation which can be defined as the method to calculate the change in rate of some quantity with respect to another let take the help of an example to explain this concept suppose a driver is driving his car with speed of 12 km/hr having acceleration of
Complete step-by-step solution:

According to the given information we have a conical funnel leaking water at the rate of
The radius of conical funnel is 10 cm i.e. r = 10 cm and altitude is 20 cm i.e. h = 20 cm
We know that the volume of the conical flask is given by
Let
As we know that the ratio of radius and height of conical flask will remain same when the flask is full of water
So,
Substituting the given values in the equation 1 we get
Differentiating the above equation both side with respect to t we get
Substituting the values in the above equation we get
Therefore the rate of water level dropping in conical flask when water starts leaking is
Note: In the above solution we used the method of differentiation which can be defined as the method to calculate the change in rate of some quantity with respect to another let take the help of an example to explain this concept suppose a driver is driving his car with speed of 12 km/hr having acceleration of
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




