
How much water should be filled in a container 21cm in height so that it appears half-filled when viewed from the top of a container?
(Refractive index of water =
A. 8 cm
B. 10.5 cm
C. 12cm
D. 14 cm

Answer
519.9k+ views
Hint – We must know the formula of
Complete step-by-step solution -
When the light travels from rarer to the denser medium it bends towards normal to the surface of the medium and when it travels from denser to the rarer medium it bends away from the normal. This phenomena of bending of light is called refraction.
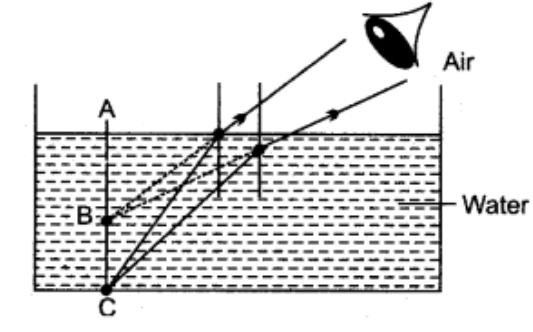
Let us say that the level of water is away from the top of the container AB by ‘x’ cm. The actual level of water would then be ‘21-x’ cm. Also, the apparent depth would be the same level ‘x’ cm.
The container will appear half filled when the appearance of point C will be in the place of B.
So, after using the formula we can say,
Hence we can say that,
Therefore, level of water required to be filled = (21-9) cm = 12cm
Hence, the correct option is C that is 12cm.
Note – We must be aware that the let quantity x is the level of water away from the top of the container and then apply the formula
Complete step-by-step solution -
When the light travels from rarer to the denser medium it bends towards normal to the surface of the medium and when it travels from denser to the rarer medium it bends away from the normal. This phenomena of bending of light is called refraction.
Let us say that the level of water is away from the top of the container AB by ‘x’ cm. The actual level of water would then be ‘21-x’ cm. Also, the apparent depth would be the same level ‘x’ cm.
The container will appear half filled when the appearance of point C will be in the place of B.
So, after using the formula we can say,
Hence we can say that,
Therefore, level of water required to be filled = (21-9) cm = 12cm
Hence, the correct option is C that is 12cm.
Note – We must be aware that the let quantity x is the level of water away from the top of the container and then apply the formula
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




