
We have to prove the theorem that if in two triangles, corresponding angles are equal then their corresponding sides are in the same ratio (or proportion) and hence the two triangles are similar.
Answer
500.1k+ views
Hint: First of all draw two triangles ABC and PQR and then mark two points D and E on the side PQ and PR such that
Complete step by step answer:
In the below figure, we have drawn two triangles ABC and PQR and we have drawn DE on PQR as follows:
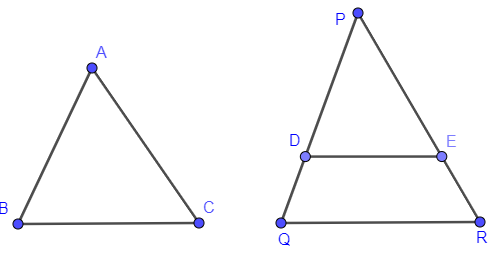
In the above figure,
It is given that corresponding angles of triangles ABC and PQR are equal so:
The below sides are equal due to its construction in such a way:
Let us consider
Then
As
It is also given that:
From eq. (1 and 3) we get,
From eq. (2 and 4) we get,
From eq. (5 and 6), we can say that DE is parallel to QR then the above angles are corresponding angles.
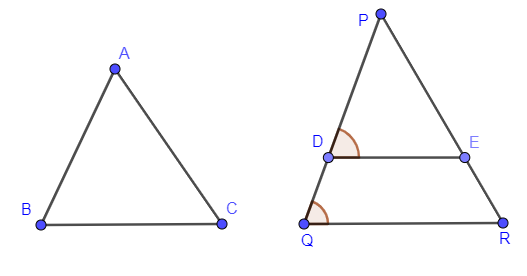
There is a theorem that if a line is parallel to one side of the triangle and intersects the other two sides in two distinct points then the other two sides are divided in the same ratio. Using this theorem in the triangle PQR,
Then, the following sides are proportional in the following way:
Taking reciprocal on both the sides of the above equation we get,
Adding 1 on both the sides we get,
Taking reciprocal on both the sides we get,
We have shown above that:
So, substituting
Similarly, we can show that:
Therefore, assemble all the proportional sides that we have shown above we get,
Since, we have shown that all the sides of the triangles ABC and PQR are proportional so:
Hence, we have shown that two triangles ABC and PQR are similar.
Note: From this solution, we have extracted some information that we can directly use in solving the other problems are as follows:
First of all the theorem itself states that if corresponding sides of two triangles are equal then the sides of the triangle are proportional to each other and hence, the two triangles are similar to each other.
The other thing is that if two triangles are congruent then corresponding part of the congruent triangles are equal.
Complete step by step answer:
In the below figure, we have drawn two triangles ABC and PQR and we have drawn DE on PQR as follows:

In the above figure,
It is given that corresponding angles of triangles ABC and PQR are equal so:
The below sides are equal due to its construction in such a way:
Let us consider
Then
As
It is also given that:
From eq. (1 and 3) we get,
From eq. (2 and 4) we get,
From eq. (5 and 6), we can say that DE is parallel to QR then the above angles are corresponding angles.

There is a theorem that if a line is parallel to one side of the triangle and intersects the other two sides in two distinct points then the other two sides are divided in the same ratio. Using this theorem in the triangle PQR,
Then, the following sides are proportional in the following way:
Taking reciprocal on both the sides of the above equation we get,
Adding 1 on both the sides we get,
Taking reciprocal on both the sides we get,
We have shown above that:
So, substituting
Similarly, we can show that:
Therefore, assemble all the proportional sides that we have shown above we get,
Since, we have shown that all the sides of the triangles ABC and PQR are proportional so:
Hence, we have shown that two triangles ABC and PQR are similar.
Note: From this solution, we have extracted some information that we can directly use in solving the other problems are as follows:
First of all the theorem itself states that if corresponding sides of two triangles are equal then the sides of the triangle are proportional to each other and hence, the two triangles are similar to each other.
The other thing is that if two triangles are congruent then corresponding part of the congruent triangles are equal.
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

What are the public facilities provided by the government? Also explain each facility




