
What do you mean by Exchange Energy?
Answer
426.3k+ views
Hint: In order to answer the above question, we will look upon the theory of the electronic configurations of atoms and its stability. We will discuss the factors affecting the stability of atoms corresponding to electronic configuration and use examples for better understanding. Finally, we will discuss exchange energy in detail and its importance in the stability of atoms. We will also derive its formulae.
Complete answer: Atoms prefer to remain in their lowest energy state. As a result, the electronic configuration of an atom is written corresponding to its lowest energy state. Atoms are stable if their electrons are in the lowest energy orbitals. Since the higher the energy of the electrons, the more excited they become, and the more unstable the atom becomes.
However, certain elements, such as copper and chromium, deviate from the standard electronic configuration. Copper and chromium should be arranged as follows:
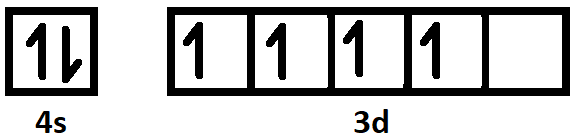

But this appears to be the wrong configuration of
Let's figure out how to configure it correctly and why it's configured that way. Before we can learn the proper way to configure it, we must first understand why it has different configurations.
(1) Half-filled and fully filled orbitals: Other configurations have less stability than half-filled and fully filled orbitals. It means that if a half-filled orbital can be obtained by disrupting only one electron, such configurations are favoured. These are, in general, the best electronic configurations for any atom. Also, since there is less shielding between the electrons in this configuration, they are highly attracted to the nucleus. As a result, in the case of half-filled and completely filled orbitals, the atom becomes more stable.
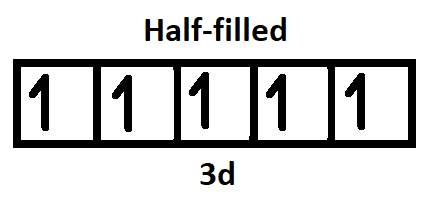
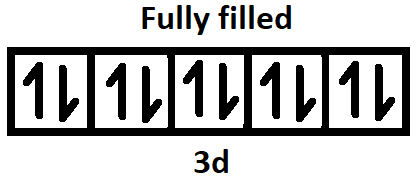
(2) Exchange energy: In general, electrons in different orbitals of the same subshell appear to swap positions. And this form of exchange results in a loss of energy known as exchange energy. The more exchanges there are, the more exchange energy is released, and thus the greater the stability. Since the number of exchanges in a half-filled and fully filled orbital is the highest, the exchange energy is also the highest, resulting in the atom's maximum stability.

As a result, exchange energy is proportional to the number of locations of electrons with the same spins that are exchanged from one orbital to another in the same subshell, and it equals
If with each exchange releases a certain definite amount of energy K, then
Where
Taking into account the concept of stability of half-filled and fully filled orbitals, we try to write the configuration of
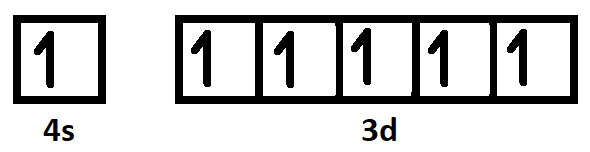

Note:
It is very important to note that the exchange of electrons having similar spins follow a specific protocol for exchange. The exchange of energy plays a vital role in the stability of an atom. The greater the exchange energy, the greater will be the release of energy and the greater will be the atomic stability. Exchange energy forms an important part of the formation of covalent bonds in many solids and is also the backbone for ferromagnetic coupling.
Complete answer: Atoms prefer to remain in their lowest energy state. As a result, the electronic configuration of an atom is written corresponding to its lowest energy state. Atoms are stable if their electrons are in the lowest energy orbitals. Since the higher the energy of the electrons, the more excited they become, and the more unstable the atom becomes.
However, certain elements, such as copper and chromium, deviate from the standard electronic configuration. Copper and chromium should be arranged as follows:
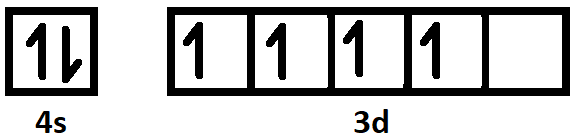

But this appears to be the wrong configuration of
Let's figure out how to configure it correctly and why it's configured that way. Before we can learn the proper way to configure it, we must first understand why it has different configurations.
(1) Half-filled and fully filled orbitals: Other configurations have less stability than half-filled and fully filled orbitals. It means that if a half-filled orbital can be obtained by disrupting only one electron, such configurations are favoured. These are, in general, the best electronic configurations for any atom. Also, since there is less shielding between the electrons in this configuration, they are highly attracted to the nucleus. As a result, in the case of half-filled and completely filled orbitals, the atom becomes more stable.


(2) Exchange energy: In general, electrons in different orbitals of the same subshell appear to swap positions. And this form of exchange results in a loss of energy known as exchange energy. The more exchanges there are, the more exchange energy is released, and thus the greater the stability. Since the number of exchanges in a half-filled and fully filled orbital is the highest, the exchange energy is also the highest, resulting in the atom's maximum stability.

As a result, exchange energy is proportional to the number of locations of electrons with the same spins that are exchanged from one orbital to another in the same subshell, and it equals
If with each exchange releases a certain definite amount of energy K, then
Where
Taking into account the concept of stability of half-filled and fully filled orbitals, we try to write the configuration of


Note:
It is very important to note that the exchange of electrons having similar spins follow a specific protocol for exchange. The exchange of energy plays a vital role in the stability of an atom. The greater the exchange energy, the greater will be the release of energy and the greater will be the atomic stability. Exchange energy forms an important part of the formation of covalent bonds in many solids and is also the backbone for ferromagnetic coupling.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




