Question

What does $e$ mean in math?

Answer 1

Hint: This type of problem is based on the concept of logarithmic and exponential function. The real number e in mathematics refers to the natural number or Euler’s number. The value of e found by experiment is 2.7182818 approximately. Functions can be defined from the number e which is called exponential function.

Complete step by step solution:
According to the question, we are asked to find what he means in mathematics.
‘e’ is a real number which is called a natural number or Euler’s number.
According to the definition of real numbers, all the rational numbers and irrational numbers are considered to be real numbers.
The value of e found by calculation is 2.7182818……
Here, we find that there are infinite numbers after the decimal. Such numbers are called irrational numbers.
Therefore, e is an irrational number which is a real number.
The approximate value of e is 2.718 which are used for calculation.
According to the definition of exponential function, \[{{e}^{x}}\] is an infinite series, which is
\[{{e}^{x}}=1+\dfrac{x}{1!}+\dfrac{{{x}^{2}}}{2!}+\dfrac{{{x}^{3}}}{3!}+\dfrac{{{x}^{4}}}{4!}+.........\infty \]
Here, the symbol ‘!’ refers to the factorial, that is \[x!=x\left( x-1 \right)\left( x-2 \right).....3.2.1\].
Now, we are asked to find the value of e.
\[\Rightarrow e=1+\dfrac{1}{1!}+\dfrac{{{1}^{2}}}{2!}+\dfrac{{{1}^{3}}}{3!}+\dfrac{{{1}^{4}}}{4!}+.........\infty \]
\[\Rightarrow e=1+\dfrac{1}{1!}+\dfrac{1}{2!}+\dfrac{1}{3!}+\dfrac{1}{4!}+.........\infty \]
On further simplification, we get
e=2. 7182818……
We can plot the graph of y=e.
Therefore, we get

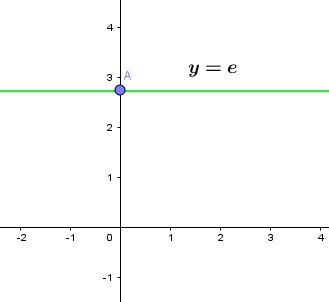
The real number e in logarithmic function plays a different role. If the base of the logarithmic function is e, then the logarithmic function turns to natural logarithm.
That is \[{{\log }_{e}}x=\ln x\].

Note: We can also represent e as \[{{e}^{1}}\].
We know the relation between logarithmic function and exponential function over x.
That is \[\ln \left( {{e}^{x}} \right)=x\].
Here, x=1.
Therefore, we get $ln(e)=1$