
What is CSC trigonometry?
Answer
417k+ views
Hint: First, we need to analyze the given information so that we are able to discuss the problem. Generally, in Mathematics, the trigonometric Identities are useful whenever trigonometric functions are involved in an expression or an equation and these identities are useful whenever expressions involving trigonometric functions need to be simplified.
Complete step-by-step solution:
Given that CSC means cosecant trigonometry which is the inverse function of the sine value which can be also represented as
Hence the cosecant is the angle of ratio length of hypotenuse and length of sides opposite angle.
Therefore,
The reciprocal identity of the trigonometric functions states that three functions can be defined as the reciprocal of the other three functions such that, secant can be defined as cosine, cosecant can be defined as the sine, and cotangent can be defined as the tangent.
Where trigonometric is the branch of mathematics that will deal with the specific function of angles and also their application in the calculation
Additional information:
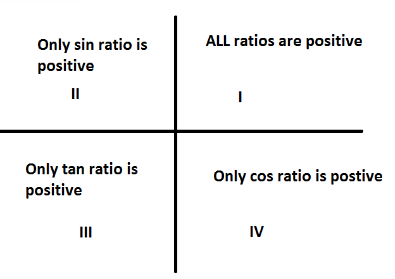
We shall analyze the given graph first.
Here in the first quadrant, all ratios are positive. In the second quadrant, only sine is positive, and in the third quadrant, only the tangent ratio is positive. And in the fourth quadrant, only the cosine ratio is positive.
When we are given
Note: The six basic trigonometric ratios are sine, cosine, tangent, cosecant, secant, and cotangent and all the fundamental trigonometric identities are derived from the six trigonometric ratios.
The trigonometric functions are those real functions that relate the angle to the ratio of two sides of a right-angled triangle.
Complete step-by-step solution:
Given that CSC means cosecant trigonometry which is the inverse function of the sine value which can be also represented as
Hence the cosecant is the angle of ratio length of hypotenuse and length of sides opposite angle.
Therefore,
The reciprocal identity of the trigonometric functions states that three functions can be defined as the reciprocal of the other three functions such that, secant can be defined as cosine, cosecant can be defined as the sine, and cotangent can be defined as the tangent.
Where trigonometric is the branch of mathematics that will deal with the specific function of angles and also their application in the calculation
Additional information:
We shall analyze the given graph first.
Here in the first quadrant, all ratios are positive. In the second quadrant, only sine is positive, and in the third quadrant, only the tangent ratio is positive. And in the fourth quadrant, only the cosine ratio is positive.

When we are given
Note: The six basic trigonometric ratios are sine, cosine, tangent, cosecant, secant, and cotangent and all the fundamental trigonometric identities are derived from the six trigonometric ratios.
The trigonometric functions are those real functions that relate the angle to the ratio of two sides of a right-angled triangle.
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

What are the public facilities provided by the government? Also explain each facility




