
What is the directrix of a parabola?
Answer
433.5k+ views
Hint: We first define what a parabola is. Its general equation can be given as
Complete step-by-step solution:
In order to answer this question, we define what a parabola is. A parabola is a curvilinear shape where any point on the curve is equidistant from a point called the focus and from a line called the directrix of the parabola. We draw a parabola whose general equation is as follows:
Here, a stands for the coordinates of the focus of the parabola. We have drawn the parabolic curve along with the directrix line as shown in the figure below.
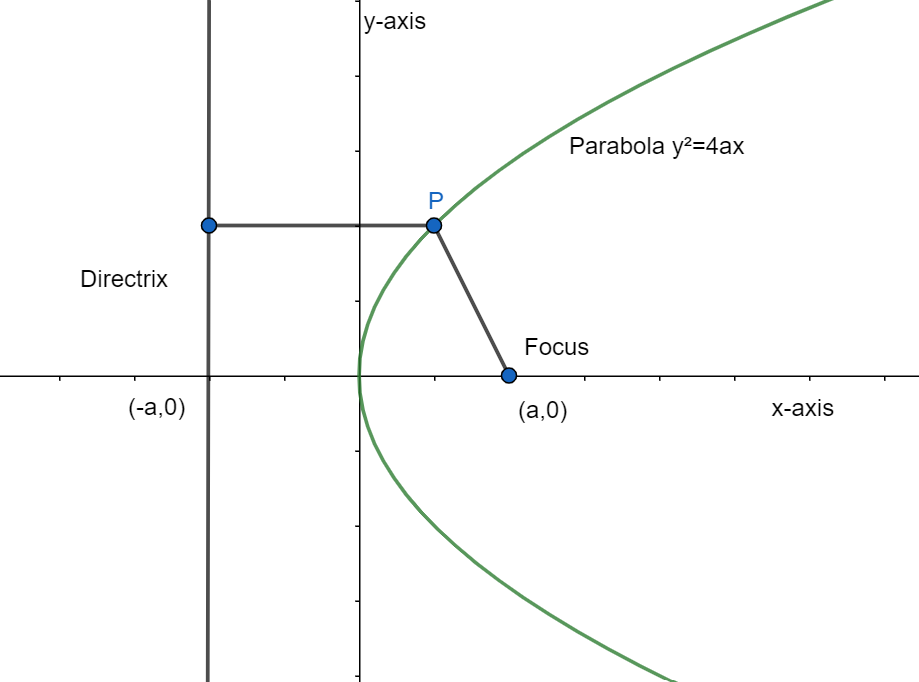
As shown in the figure, the directrix is a straight line parallel to the y-axis for the parabola with a general equation of the form
Hence, the directrix is a line parallel to the y-axis and is important for defining the parabolic curve.
Note: It is important to know the basic concepts of parabola and its definition. This will help us solve such questions. One important point to note is that the directrix is always perpendicular to the axis of symmetry of the parabola which is the x-axis in this case.
Complete step-by-step solution:
In order to answer this question, we define what a parabola is. A parabola is a curvilinear shape where any point on the curve is equidistant from a point called the focus and from a line called the directrix of the parabola. We draw a parabola whose general equation is as follows:
Here, a stands for the coordinates of the focus of the parabola. We have drawn the parabolic curve along with the directrix line as shown in the figure below.

As shown in the figure, the directrix is a straight line parallel to the y-axis for the parabola with a general equation of the form
Hence, the directrix is a line parallel to the y-axis and is important for defining the parabolic curve.
Note: It is important to know the basic concepts of parabola and its definition. This will help us solve such questions. One important point to note is that the directrix is always perpendicular to the axis of symmetry of the parabola which is the x-axis in this case.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




