
What is the domain of
Answer
439.2k+ views
Hint: We can define that, the domain of a function is the complete step of possible values of the independent variable. So, we can say that the domain is the set of all possible ‘x’ values which will make the function ‘work’ and will give the output of ‘y’ as a real number. We remember that,
Complete step by step answer:
The domain of the expression is all real numbers except where the expression is undefined, which means there are no real numbers that make the expression undefined. It is given that,
As we know that,
It means the
We will now find the domain by
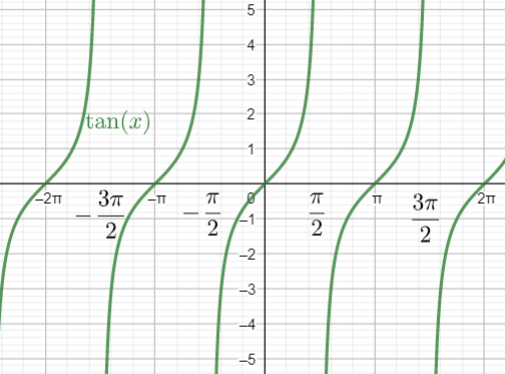
The trigonometric values of
The curve of
Therefore, the domain of
Domain:
Note: We must know the trigonometry concepts to solve this question. The domain where the x value ranges and the range where the y value rages. Here the function is a form of trigonometric function.
Complete step by step answer:
The domain of the expression is all real numbers except where the expression is undefined, which means there are no real numbers that make the expression undefined. It is given that,
As we know that,
It means the
We will now find the domain by

The trigonometric values of
| 0 | |||||
| 0 | 1 | -1 |
The curve of
Therefore, the domain of
Domain:
Note: We must know the trigonometry concepts to solve this question. The domain where the x value ranges and the range where the y value rages. Here the function is a form of trigonometric function.
Recently Updated Pages
The number in which 7 has the greatest place value class 7 maths CBSE

Find the additive inverse of the following integers class 7 maths CBSE

Ali and Beth divide 280 in the ratio 25 How much does class 7 maths CBSE

How do you convert 0789 789 repeating to a fractio class 7 maths CBSE

If the cost of x meters of wires is d rupees then the class 7 maths CBSE

Find the value of x such that 5x 12 18 5x-class-7-maths-CBSE

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




