Question

What is the hypotenuse – leg theorem?

Answer 1

Hint: First of all we will understand the hypotenuse – leg theorem and what it is used for. Now, we will take examples of two right angle triangles with some of the given assumptions and we will try to prove them congruent using the hypotenuse – leg theorem. Also we will see its relation with R.H.S congruence criteria.

Complete answer:
Here we are asked to describe the hypotenuse – leg theorem. So, we need to understand the statement of the theorem and then we need to see its use.
Now, the hypotenuse – leg theorem is used to prove if two given right angle triangles are congruent or not. The theorem states that ‘if the hypotenuse and one side of a right angle triangle is equal to the hypotenuse and one side of the other triangle then the two right angle triangles are congruent to each other’.
Let us consider an example: - here we are assuming an isosceles triangle ABC with sides AB = AC, we draw a perpendicular AD from the vertex A to the side BC. We need to prove that the right triangles ABD and ACD are congruent.

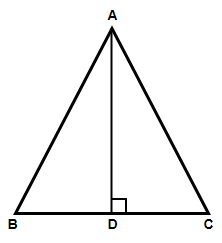
Now, in right triangles ABD with ACD we have,
AB = AC (triangle ABC is isosceles)
AD =AD (common side)
Clearly we can see that AB is the hypotenuse of triangle ABD and similarly AC is the hypotenuse of the triangle ACD while AD is the side common in them. So we can say that the hypotenuse and one side of triangle ABD is equal to the hypotenuse and the other side of the triangle ACD. Therefore, the two right triangles are congruent by the hypotenuse – leg theorem.

Note: Note that the hypotenuse – leg theorem is also called the R.H.S congruence criteria. R.H.S is a congruence criteria in which R, H and S stands for right angle, hypotenuse and side respectively. It is used to prove two triangles congruent when they have a right angle, their hypotenuses are of equal length and one of the remaining two sides are also equal.