
What is the MOED of $H{e_2}$ molecule?
Answer
450.6k+ views
Hint: As we know that molecular orbital theory assumes that in molecules the atomic orbitals lose their identity and the electrons in molecules are present in new orbitals called molecular orbitals. Molecular orbitals energy diagrams show the relative energies of molecular orbitals.
Complete step by step answer:
The molecular orbital theory assumes that the atomic orbitals in molecules lose their identity and the electrons in these molecules are present in new orbitals which are commonly called molecular orbitals. When two atomic orbitals combine, formation of two molecular orbitals take place. The molecular orbitals formed by the overlapping of atomic orbitals is called bonding molecular orbital (BMO) and the molecular orbitals formed by the subtraction overlap of atomic orbitals is called anti-bonding molecular orbitals (ABMO).
Energy level diagrams for molecular orbitals can be drawn using the increasing order of energies which is given as:
$\sigma 1s < {\sigma ^*}1s < \sigma 2s < {\sigma ^*}2s < \sigma 2{p_z} < [\pi 2{p_x} = \pi 2{p_y}] < [{\pi ^*}2{p_x} = {\pi ^*}2{p_y}] < {\sigma ^*}2{p_z}$
As we know that Helium has an atomic number $2$. So the electronic configuration is $1{s^2}$and two atoms of helium are present so four electrons will be there in total which will accommodate the $\sigma 1s$ and ${\sigma ^*}1s$ molecular orbitals. Thus, we can draw the molecular orbital energy diagram as:
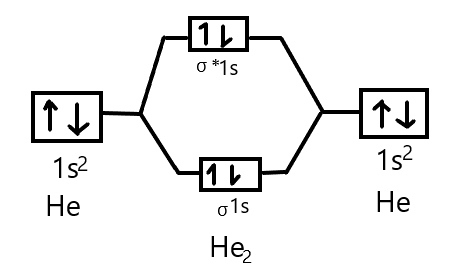
The stability of molecules can be determined by its bond order which is defined as the half the difference between the number of electrons in bonding molecular orbitals and the number of electrons in antibonding molecular orbitals. It is given as:
$Bond\;order = \dfrac{1}{2}[{N_b} - {N_a}]$
And we got from the diagram that Helium has two electrons in its bonding molecular orbital and two electrons in its anti-bonding molecular orbital. So its bond order will be:
$Bond\;order = \dfrac{1}{2}[2 - 2] = 0$
So, the bond comes out to be zero, therefore the $H{e_2}$ molecule is unstable and does not exist.
Note: Remember if the value of bond order is positive, it indicated that the molecule is stable and if the value of bond order is negative or zero then it suggests that the molecule is highly unstable and is not formed. The dissociation energy is directly proportional to the bond order of the molecule. Greater the bond order, greater will be the bond dissociation energy.
Complete step by step answer:
The molecular orbital theory assumes that the atomic orbitals in molecules lose their identity and the electrons in these molecules are present in new orbitals which are commonly called molecular orbitals. When two atomic orbitals combine, formation of two molecular orbitals take place. The molecular orbitals formed by the overlapping of atomic orbitals is called bonding molecular orbital (BMO) and the molecular orbitals formed by the subtraction overlap of atomic orbitals is called anti-bonding molecular orbitals (ABMO).
Energy level diagrams for molecular orbitals can be drawn using the increasing order of energies which is given as:
$\sigma 1s < {\sigma ^*}1s < \sigma 2s < {\sigma ^*}2s < \sigma 2{p_z} < [\pi 2{p_x} = \pi 2{p_y}] < [{\pi ^*}2{p_x} = {\pi ^*}2{p_y}] < {\sigma ^*}2{p_z}$
As we know that Helium has an atomic number $2$. So the electronic configuration is $1{s^2}$and two atoms of helium are present so four electrons will be there in total which will accommodate the $\sigma 1s$ and ${\sigma ^*}1s$ molecular orbitals. Thus, we can draw the molecular orbital energy diagram as:

The stability of molecules can be determined by its bond order which is defined as the half the difference between the number of electrons in bonding molecular orbitals and the number of electrons in antibonding molecular orbitals. It is given as:
$Bond\;order = \dfrac{1}{2}[{N_b} - {N_a}]$
And we got from the diagram that Helium has two electrons in its bonding molecular orbital and two electrons in its anti-bonding molecular orbital. So its bond order will be:
$Bond\;order = \dfrac{1}{2}[2 - 2] = 0$
So, the bond comes out to be zero, therefore the $H{e_2}$ molecule is unstable and does not exist.
Note: Remember if the value of bond order is positive, it indicated that the molecule is stable and if the value of bond order is negative or zero then it suggests that the molecule is highly unstable and is not formed. The dissociation energy is directly proportional to the bond order of the molecule. Greater the bond order, greater will be the bond dissociation energy.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
10 examples of friction in our daily life

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

Pigmented layer in the eye is called as a Cornea b class 11 biology CBSE

State the laws of reflection of light




