
What is the range of
Answer
404.4k+ views
Hint: We are given the function
Complete step-by-step answer:
We first see What does domain stand for?
Domain is basically the set of all values that qualify as we input them in a function.
The range of a function is the set of all outputs of a function when domain values are substituted in the function.
Let us consider a function
Now, plotting the graph of the function
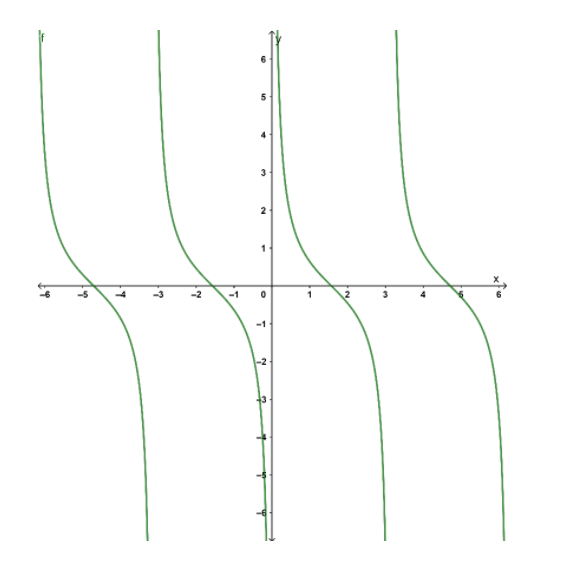
From the graph, we see that the graph is not continuous, which means that the function is not defined on the points where the graph breaks. The points which take the value of
Since we need to find out the range of the function
Hence, we see that
So, the range of
Or, we can write it as
Note: While finding the range and domain of the function, we need to check where the function is not defined and for that we need to consider each and every point on the real line (Since we are dealing with real numbers only). Basically, for finding the range of a function
Complete step-by-step answer:
We first see What does domain stand for?
Domain is basically the set of all values that qualify as we input them in a function.
The range of a function is the set of all outputs of a function when domain values are substituted in the function.
Let us consider a function
Now, plotting the graph of the function

From the graph, we see that the graph is not continuous, which means that the function is not defined on the points where the graph breaks. The points which take the value of
Since we need to find out the range of the function
Hence, we see that
So, the range of
Or, we can write it as
Note: While finding the range and domain of the function, we need to check where the function is not defined and for that we need to consider each and every point on the real line (Since we are dealing with real numbers only). Basically, for finding the range of a function
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




