
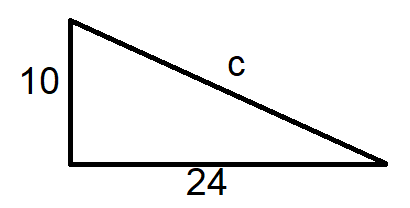
What is the value of

Answer
436.2k+ views
Hint: We first describe the Pythagorean identity with respect to the right-angle triangle. We use the formula of
Complete step by step solution:
The Pythagorean identity is about the trigonometric identity that is used in case of right-angle triangles.
The Pythagorean trigonometric identity, also called simply the Pythagorean identity, is an identity expressing the Pythagorean theorem in terms of trigonometric functions. Along with the sum-of-angles formulae, it is one of the basic relations between the sine and cosine functions.
We express it for
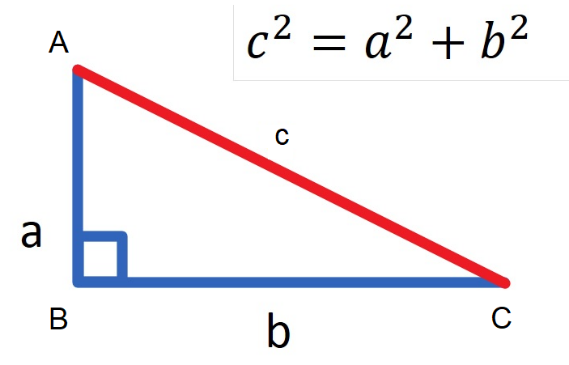
We have the expression for the triangle as
For
Applying the rule, we get
We can also express it with respect to the angle
The equation
With respect to the angle
Replacing the values, we get
There are many reformed versions of the formula
Dividing with
Dividing with
For the given image we have 10 as height and 24 as base.
Therefore,
Taking square root, we get
We have the length of the hypotenuse as 26 units.
Note: The concept of Pythagorean identity is similar for both angles and sides. The representation of the triangle has to be for the right-angle triangle.
The figure shows how the sign of the sine function varies as the angle changes quadrant.
Complete step by step solution:
The Pythagorean identity is about the trigonometric identity that is used in case of right-angle triangles.
The Pythagorean trigonometric identity, also called simply the Pythagorean identity, is an identity expressing the Pythagorean theorem in terms of trigonometric functions. Along with the sum-of-angles formulae, it is one of the basic relations between the sine and cosine functions.
We express it for

We have the expression for the triangle as
For
Applying the rule, we get
We can also express it with respect to the angle
The equation
With respect to the angle
Replacing the values, we get
There are many reformed versions of the formula
Dividing with
Dividing with
For the given image we have 10 as height and 24 as base.
Therefore,
Taking square root, we get
We have the length of the hypotenuse as 26 units.
Note: The concept of Pythagorean identity is similar for both angles and sides. The representation of the triangle has to be for the right-angle triangle.
The figure shows how the sign of the sine function varies as the angle changes quadrant.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




