
What is the value of
Answer
407.4k+ views
Hint: When we work with triangles in trigonometry, then our task becomes easier and it becomes easy for us to solve our question. The Cartesian system should be known to you and you should also know that there are six functions for angles of trigonometry which are sine, cosine, secant, cosecant, tangent, cotangent.
Complete step-by-step answer:
Trigonometry is the study of measurements of triangles which deals with the length, height, and angles of the triangle. The trigonometry functions have a different application in the real world. In various fields like engineering, architecture, satellite navigation, development of waves, etc.
Trigonometry is the branch of mathematics that deals with various functions of angles and there are six different functions of angles which are sine, cosine, tangent, cotangent, secant, and cosecant.
All the different angles of trigonometry are there in the Cartesian plane which consists of four quadrants. In the first quadrant of the Cartesian system, all the angles will have a positive value. In the second quadrant, the value of angles of sine and cosecant will be positive. In the third quadrant, the value of tangent and cotangent will be positive, and in the fourth quadrant, cosine and secant will be positive.
The value of
There is a unit circle in which the cartesian plane is divided into four quadrants.
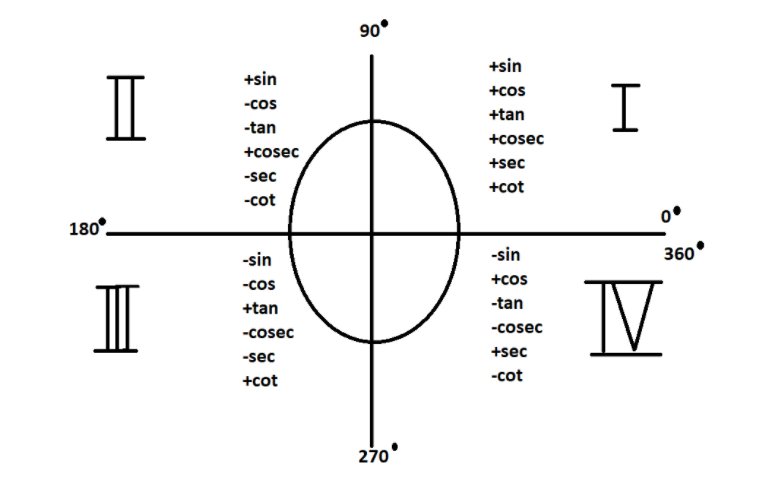
From the above diagram we can figure out the following:
So to find the value of
We know that the value of
On Substituting the obtained value in the above relation, we get
So, the value of
So, the correct answer is “-1”.
Note: The angles of trigonometry are associated with the base, height, and hypotenuse of a triangle, like a sine angle represents the ratio between height and hypotenuse of the triangle. Cosine angle represents the ratio between the base and hypotenuse of a triangle whereas tangent angle represents the ratio between height and base of the triangle.
Complete step-by-step answer:
Trigonometry is the study of measurements of triangles which deals with the length, height, and angles of the triangle. The trigonometry functions have a different application in the real world. In various fields like engineering, architecture, satellite navigation, development of waves, etc.
Trigonometry is the branch of mathematics that deals with various functions of angles and there are six different functions of angles which are sine, cosine, tangent, cotangent, secant, and cosecant.
All the different angles of trigonometry are there in the Cartesian plane which consists of four quadrants. In the first quadrant of the Cartesian system, all the angles will have a positive value. In the second quadrant, the value of angles of sine and cosecant will be positive. In the third quadrant, the value of tangent and cotangent will be positive, and in the fourth quadrant, cosine and secant will be positive.
The value of
There is a unit circle in which the cartesian plane is divided into four quadrants.

From the above diagram we can figure out the following:
So to find the value of
We know that the value of
On Substituting the obtained value in the above relation, we get
So, the value of
So, the correct answer is “-1”.
Note: The angles of trigonometry are associated with the base, height, and hypotenuse of a triangle, like a sine angle represents the ratio between height and hypotenuse of the triangle. Cosine angle represents the ratio between the base and hypotenuse of a triangle whereas tangent angle represents the ratio between height and base of the triangle.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




