
What is the value of
Answer
432.9k+ views
Hint: Here the question has a trigonometric function, we have to find the exact value of
Complete step-by-step answer:
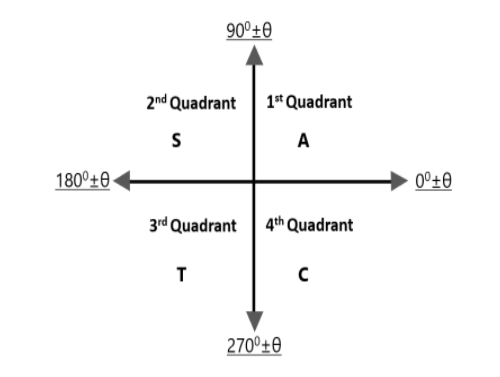
‘ASTC’ abbreviated as 'all sin cos tan' rule in trigonometry. The first letter of the first word in this phrase is 'A'. This may be taken to indicate that all trigonometric ratios in the first quadrant are positive. The first letter of the second word ‘S’ indicates that sine and its reciprocal cosecant are positive in the 2nd quadrant. The first letter of the third word ‘T’ indicates that tangent and its reciprocal cotangent is positive in the third quadrant. The first letter of the last word ‘C’ indicates that cosine and its reciprocal secant are positive in the fourth quadrant.
Consider the given question.
We have to find the value of
First, solve the value of
The value of
The angle
Now Consider,
By ASTC rule of trigonometry, the angle
We know that the exact value of
Or
Consider
By ASTC rule of trigonometry, the angle
We know that the exact value of
Now, we know the value of
As we know cosine or cos is an even function. This means that
Substitute the value of
Hence, the exact value of
So, the correct answer is “-1”.
Note: Before solving questions of this kind we should be fully aware of all the trigonometric functions and their values. We should note that the value of
Complete step-by-step answer:

‘ASTC’ abbreviated as 'all sin cos tan' rule in trigonometry. The first letter of the first word in this phrase is 'A'. This may be taken to indicate that all trigonometric ratios in the first quadrant are positive. The first letter of the second word ‘S’ indicates that sine and its reciprocal cosecant are positive in the 2nd quadrant. The first letter of the third word ‘T’ indicates that tangent and its reciprocal cotangent is positive in the third quadrant. The first letter of the last word ‘C’ indicates that cosine and its reciprocal secant are positive in the fourth quadrant.
Consider the given question.
We have to find the value of
First, solve the value of
The value of
The angle
Now Consider,
By ASTC rule of trigonometry, the angle
We know that the exact value of
Or
Consider
By ASTC rule of trigonometry, the angle
We know that the exact value of
Now, we know the value of
As we know cosine or cos is an even function. This means that
Substitute the value of
Hence, the exact value of
So, the correct answer is “-1”.
Note: Before solving questions of this kind we should be fully aware of all the trigonometric functions and their values. We should note that the value of
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




