
Which of the following graphs represents Boyles’s law? This question has multiple correct options.

Answer
515.4k+ views
1 likes
Hint: We should recall the concepts of Boyle’s law. We should know the relationship between pressure and volume. Boyles’s law tells us about the change in volume when pressure is exerted by gas. It can be inversely proportional or directly proportional.
Complete step by step answer:
So, if we recall Boyle's law, we come to know that Boyle’s law is a gas law which states that the pressure exerted by a gas (of a given mass, kept at a constant temperature) is inversely proportional to the volume occupied by it. In other words, we can say that the pressure and volume of a gas are inversely proportional to each other as long as the temperature and the quantity of gas are kept constant.
For a gas, we can tell the relationship between volume and pressure (at constant mass and temperature). It can be mathematically expressed as follows:
P ∝ (
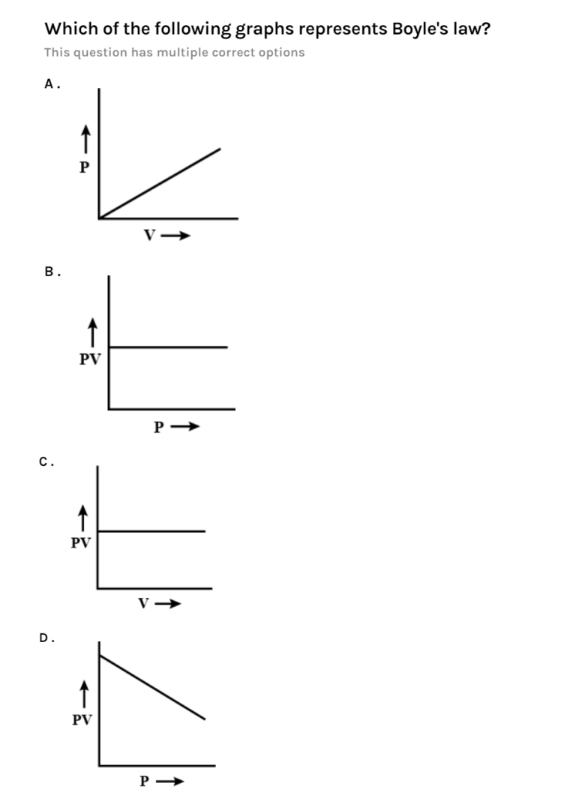
From the above equation, we came to know that the product of pressure and volume is always equals to constant k. So, if we observe the graph we came to know that the options B and C represent Boyle's law as PV= constant.
In both the options B and C, PV is behaving as constant just as in the equation. Now we will check other options also.
If we take option A, we see that as pressure is increasing proportional to volume. Graph is showing as pressure increases volume also increases uniformly. This graph is not a correct representation of Boyle’s law.
If we represent the equation from the graph represented in option A. It will look like this,
If we now come to option D, the graph in it states that the product of pressure and volume is not constant. If we look at the graph it is looking like a product of pressure and volume is decreasing with increasing pressure. This is not Boyle’s law. Because Boyle’s law states that the product of pressure and volume is constant.
So, option D is incorrect.
From the above discussion we came to know that the correct options of this question are option B and C. this is because Boyle's law states that product of pressure and volume is constant and these graphs also say that product of pressure and volume is constant whether we increase pressure or volume it will always remain constant.
So, option B and C are correct.
Additional information:
There is a derivation of Boyle’s law that we should learn:
From Boyle's law we study, we can say that any change in the volume occupied by a gas (at constant quantity and temperature) will result in a change in the pressure exerted by it. In other words, we can say that the product of the initial pressure and the initial volume of a gas are equal to the product of its final pressure and final volume (at constant temperature and number of moles). This law can be expressed mathematically as follows:
Where,
We had obtained this expression from the pressure-volume relationship given by Boyle’s law. For a fixed amount of gas kept at a constant temperature, PV = k. Therefore,
So,
We can learn more about Boyle’s law by taking one example : when we squeeze a filled balloon, the volume occupied by the air inside the balloon decreases. This is accompanied by an increase in the pressure exerted by the air on the balloon, as a consequence of Boyle’s law. As the balloon is squeezed further, the increasing pressure eventually pops it.
Note: There is one application of Boyle’s law where its understanding is beneficial. We understand this by taking an example of scuba diver. If a scuba diver rapidly comes back from a deep zone towards the surface of the water, the decrease in the pressure can cause the gas molecules in his/her body to expand. These gas bubbles can go on to cause damage to the diver’s organs and can also result in death.
Complete step by step answer:
So, if we recall Boyle's law, we come to know that Boyle’s law is a gas law which states that the pressure exerted by a gas (of a given mass, kept at a constant temperature) is inversely proportional to the volume occupied by it. In other words, we can say that the pressure and volume of a gas are inversely proportional to each other as long as the temperature and the quantity of gas are kept constant.
For a gas, we can tell the relationship between volume and pressure (at constant mass and temperature). It can be mathematically expressed as follows:
P ∝ (
From the above equation, we came to know that the product of pressure and volume is always equals to constant k. So, if we observe the graph we came to know that the options B and C represent Boyle's law as PV= constant.
In both the options B and C, PV is behaving as constant just as in the equation. Now we will check other options also.
If we take option A, we see that as pressure is increasing proportional to volume. Graph is showing as pressure increases volume also increases uniformly. This graph is not a correct representation of Boyle’s law.
If we represent the equation from the graph represented in option A. It will look like this,
If we now come to option D, the graph in it states that the product of pressure and volume is not constant. If we look at the graph it is looking like a product of pressure and volume is decreasing with increasing pressure. This is not Boyle’s law. Because Boyle’s law states that the product of pressure and volume is constant.
So, option D is incorrect.
From the above discussion we came to know that the correct options of this question are option B and C. this is because Boyle's law states that product of pressure and volume is constant and these graphs also say that product of pressure and volume is constant whether we increase pressure or volume it will always remain constant.
So, option B and C are correct.
Additional information:
There is a derivation of Boyle’s law that we should learn:
From Boyle's law we study, we can say that any change in the volume occupied by a gas (at constant quantity and temperature) will result in a change in the pressure exerted by it. In other words, we can say that the product of the initial pressure and the initial volume of a gas are equal to the product of its final pressure and final volume (at constant temperature and number of moles). This law can be expressed mathematically as follows:
Where,
We had obtained this expression from the pressure-volume relationship given by Boyle’s law. For a fixed amount of gas kept at a constant temperature, PV = k. Therefore,
So,
We can learn more about Boyle’s law by taking one example : when we squeeze a filled balloon, the volume occupied by the air inside the balloon decreases. This is accompanied by an increase in the pressure exerted by the air on the balloon, as a consequence of Boyle’s law. As the balloon is squeezed further, the increasing pressure eventually pops it.
Note: There is one application of Boyle’s law where its understanding is beneficial. We understand this by taking an example of scuba diver. If a scuba diver rapidly comes back from a deep zone towards the surface of the water, the decrease in the pressure can cause the gas molecules in his/her body to expand. These gas bubbles can go on to cause damage to the diver’s organs and can also result in death.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹31,500 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




