Question

Which value of tan is 2?

Answer 1

Hint: We explain the function $arc\tan \left( x \right)$. We express the inverse function of tan in the form of $arc\tan \left( x \right)={{\tan }^{-1}}x$. We draw the graph of $arc\tan \left( x \right)$ and the line $x=2$ to find the intersection point as the solution.

Complete step by step answer:
The given expression is the inverse function of trigonometric ratio tan which gives us the value. The arcus function represents the angle which on ratio tan gives the value. So, $arc\tan \left( x \right)={{\tan }^{-1}}x$. If $arc\tan \left( x \right)=\alpha $ then we can say $\tan \alpha =x$.Each of the trigonometric functions is periodic in the real part of its argument, running through all its values twice in each interval of $2\pi $.

The general solution for that value where $\tan \alpha =x$ will be $n\pi +\alpha ,n\in \mathbb{Z}$. But for $arc\tan \left( x \right)$, we won’t find the general solution. We use the principal value. For the ratio tan we have $-\dfrac{\pi }{2}\le arc\tan \left( x \right)\le \dfrac{\pi }{2}$. The graph of the function is

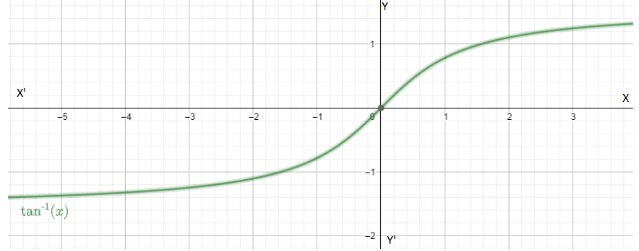
$arc\tan \left( x \right)=\alpha $ gives the angle $\alpha $ behind the ratio.
We now place the value of $x=2$ in the function of $arc\tan \left( x \right)$.
Let the angle be $\theta $ for which $arc\tan \left( 2 \right)=\theta $. This gives $\tan \theta =2$.
Putting the value in the graph of $arc\tan \left( x \right)$, we get $\theta =63.43$.
For this we take the line of $x=2$ and see the intersection of the line with the graph $arc\tan \left( x \right)$.

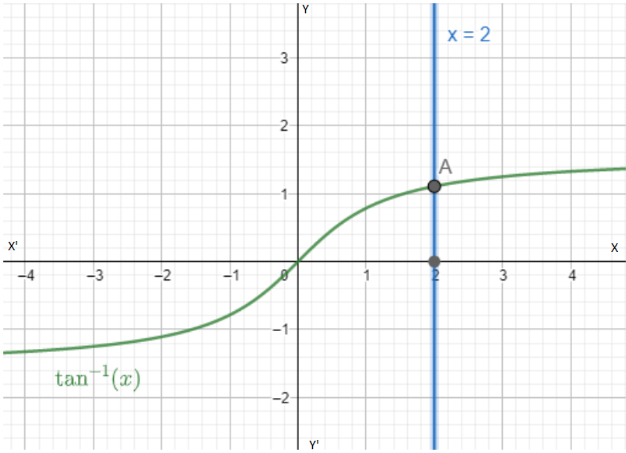
Therefore, the value of $arc\tan \left( 2 \right)$ is ${{63.43}^{\circ }}$.

Note: First note that the value 2 looks suspiciously like it was intended to be an angle but the argument of the $arc\tan \left( x \right)$ function is not an angle. The representation will be the right-angle triangle with base 1 and height 2 and the angle being $\theta $.