Question

Wien’s displacement law fails at
A. Low temperature
B. High temperature
C. Short wavelength
D. Long wavelength

Answer 1

Hint: The Wien’s displacement law is a theory related to thermodynamics. We can recall that the Wien’s displacement law gives us a relation between the peak wavelength in a blackbody spectrum and the temperature at which this peak occurs. It is essential to know that when temperature is increased, the wavelength at which the maximum Intensity is obtained becomes lesser than the value at lower temperature. 

Complete step by step solution:
Let us begin with understanding what a black body is,
A black body is a body that absorbs all the radiations that fall on it and emits radiations of all the possible wavelengths and frequencies.
When a body emits radiations, we try to know the intensity of the radiations. While Intensity tells us the quantity of the radiations or in simple words tells us how bright the light is.
However, in the case of blackbody It is observed that at a given temperature, as the wavelength is increased, the intensity increases initially, reaches a maximum value, and then goes on decreasing.

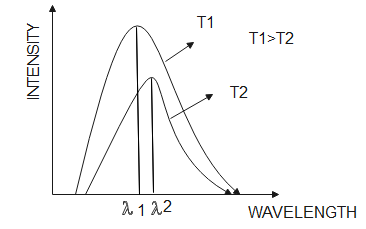
The wavelength at which the intensity is maximum is denoted as \[{{\lambda }_{\max }}\].
It is also found that as the temperature is increased, the wavelength at which the maximum intensity is obtained becomes lesser than the value at a lower temperature. 
But as per the Wien’s displacement law, there exists inverse relation between the maximum wavelength emitted by the blackbody and the temperature, which is given by,
\[{{\lambda }_{\max }}\propto \dfrac{1}{T}\]
Which means for all high wavelengths the temperature must be very low.
However, we have to know that at very low temperatures a continuous Wein’s curve cannot be obtained. Hence Wien’s displacement law fails at long wavelengths.
Therefore, option D is the correct answer.</b>

Note: Wein’s displacement law establishes a relation between \[{{\lambda }_{\max }}\] and T which is given by,
\[{{\lambda }_{\max }}T=b\]
Where, 
b is Wein’s displacement constant. 
It is also important to know that Wein’s displacement law has nothing to do with displacement.