
Work done by a centripetal force:
(A) Increase by decrease in radius of the circle.
(B) Decrease by increasing the radius of the circle.
(C) Increase by increasing mass of the body.
(D) Is always zero.
Answer
449.1k+ views
Hint :To solve this problem we should know about the basic concept of work.
Work: when force is applied on an object and it is displaced from its position in any direction. Then we can say that some work has been done.
Mathematically work will be written as:
Here,
Centripetal force: A force acting on a body to keep it moving in a circular path.
Complete Step By Step Answer:
As we know that the centripetal force causes the body to rotate in circular orbit and it acts in outward direction and displacement will be in perpendicular direction to the centripetal force.
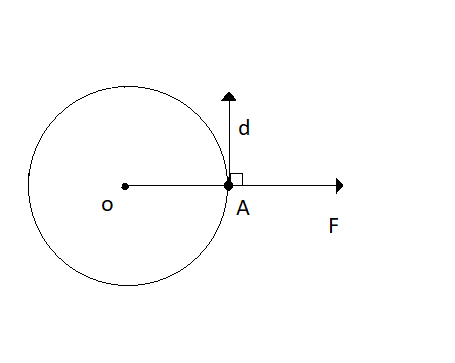
As given in the figure
As we can see from the figure, the angle between direction force and displacement will be
From the formula of force:
We get,
Hence we can observe from the above the work done by centripetal force will always be zero.
So, option (d) will be correct.
Note :
Force: A force is push and pull act upon an object which causes change in speed, direction or shape. It can be positive, negative or zero.
Displacement: the dislocation of an object from its mean position in any direction with respect to its mean position. It can be negative, positive or zero. But we know that distance can never be a negative quantity.
Centripetal force only came into existence only when circular motion existed.
Work: when force is applied on an object and it is displaced from its position in any direction. Then we can say that some work has been done.
Mathematically work will be written as:
Here,
Centripetal force: A force acting on a body to keep it moving in a circular path.
Complete Step By Step Answer:
As we know that the centripetal force causes the body to rotate in circular orbit and it acts in outward direction and displacement will be in perpendicular direction to the centripetal force.

As given in the figure
As we can see from the figure, the angle between direction force and displacement will be
From the formula of force:
We get,
Hence we can observe from the above the work done by centripetal force will always be zero.
So, option (d) will be correct.
Note :
Force: A force is push and pull act upon an object which causes change in speed, direction or shape. It can be positive, negative or zero.
Displacement: the dislocation of an object from its mean position in any direction with respect to its mean position. It can be negative, positive or zero. But we know that distance can never be a negative quantity.
Centripetal force only came into existence only when circular motion existed.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




