
Write a rational number and its opposite in the decimal form. How do you know that these are opposite rational numbers? Write a rational number and its opposite in the fraction form.
Answer
492.6k+ views
Hint:Rational numbers are the numbers which can be expressed as \[\dfrac{p}{q}\] ratio, where \[p\], \[q\] are integers and \[q \ne 0\]. The opposite of a number is the same distance from 0 as the number itself, but on the other side of 0 on a number line.
Complete step-by-step answer:
We need to first write a rational number.
Also we have to find its opposite in the decimal form.
Let us the take the rational number \[\dfrac{1}{2}\] (It is a rational number because we can expressed it as \[\dfrac{p}{q}\] ratio, where \[p\], \[q\] are integers and \[q \ne 0\]).
The decimal form of this number is \[0.5\] .
It’s opposite in the decimal form is \[ - 0.5\].
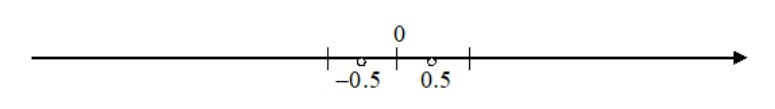
In the diagram, \[0.5\] is the opposite of \[ - 0.5\], and \[ - 0.5\] is the opposite of \[0.5\]. The distance from \[0.5\] to \[0\] is \[0.5\], and the distance from\[ - 0.5\] to \[0\] is \[0.5\]; this distance to \[0\] is the same for both \[0.5\] and \[ - 0.5\]. The absolute value of a number is its distance from \[0\] on a number line.
Let us take the rational number \[\dfrac{3}{5}\] , it’s opposite in the fraction form is \[ - \dfrac{3}{5}\].
Note:
Whole numbers: Whole numbers are simply the numbers \[0,\;{\text{ }}1,{\text{ }}2,\,{\text{ }}3,{\text{ }}4,{\text{ }}5,\,{\text{ }}6,\;....\]
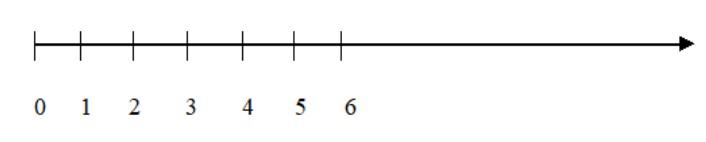
Integers: Integers are like whole numbers, but they also include negative numbers but still no fractions allowed.
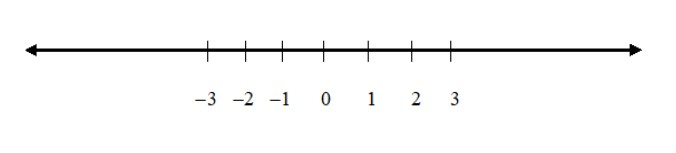
So integers can be negative {\[ - 1, - 2, - 3, - 4, - 5, - 6,....\]}, positive {\[1,2,3,4,5,6,....\]} or zero {\[0\]}.
Rational number:Rational numbers are the numbers which can be expressed as \[\dfrac{p}{q}\] ratio, where \[p\], \[q\] are integers and \[q \ne 0\].
Complete step-by-step answer:
We need to first write a rational number.
Also we have to find its opposite in the decimal form.
Let us the take the rational number \[\dfrac{1}{2}\] (It is a rational number because we can expressed it as \[\dfrac{p}{q}\] ratio, where \[p\], \[q\] are integers and \[q \ne 0\]).
The decimal form of this number is \[0.5\] .
It’s opposite in the decimal form is \[ - 0.5\].
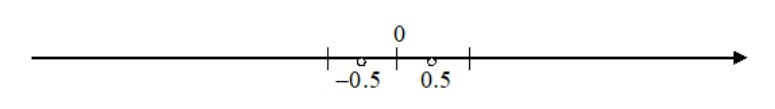
In the diagram, \[0.5\] is the opposite of \[ - 0.5\], and \[ - 0.5\] is the opposite of \[0.5\]. The distance from \[0.5\] to \[0\] is \[0.5\], and the distance from\[ - 0.5\] to \[0\] is \[0.5\]; this distance to \[0\] is the same for both \[0.5\] and \[ - 0.5\]. The absolute value of a number is its distance from \[0\] on a number line.
Let us take the rational number \[\dfrac{3}{5}\] , it’s opposite in the fraction form is \[ - \dfrac{3}{5}\].
Note:
Whole numbers: Whole numbers are simply the numbers \[0,\;{\text{ }}1,{\text{ }}2,\,{\text{ }}3,{\text{ }}4,{\text{ }}5,\,{\text{ }}6,\;....\]
Integers: Integers are like whole numbers, but they also include negative numbers but still no fractions allowed.
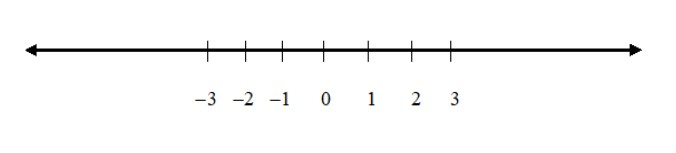
So integers can be negative {\[ - 1, - 2, - 3, - 4, - 5, - 6,....\]}, positive {\[1,2,3,4,5,6,....\]} or zero {\[0\]}.
Rational number:Rational numbers are the numbers which can be expressed as \[\dfrac{p}{q}\] ratio, where \[p\], \[q\] are integers and \[q \ne 0\].
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
Truly whole mankind is one was declared by the Kannada class 10 social science CBSE

Explain the three major features of the shiwaliks class 10 social science CBSE

Distinguish between the reserved forests and protected class 10 biology CBSE

What is the corona radiata penetrating enzyme class 10 biology CBSE

What are the public facilities provided by the government? Also explain each facility

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths




