
Write the coordinates of the vertices of a rectangle whose length and breadth are 5 units and 3 units, respectively. One vertex at the origin, the longer side lies on the x-axis and one of the vertices lie in the third quadrant.
(A). (0,0), (5,0), (0,-3) & (5,-3)
(B). (0,0), (0,-5), (3,0) & (3,-5)
(C). (0,0), (-5,0), (0,-3) & (-5,-3)
(D). (0,0), (5,0), (0,3) & (5,3)
Answer
519.6k+ views
Hint: Use the property of the rectangle that opposite sides are equal and the angle between adjacent sides is equal to
Complete step-by-step solution -
Now according to the question, one of the vertices is the origin, i.e., one of the vertices of the rectangle has coordinates (0,0). Now let us draw a diagram to check all the possible cases of rectangles which have one of their vertices to be the origin and the longer side of the rectangle lying along the x-axis.
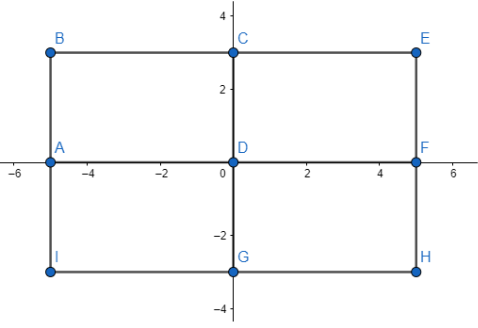
Now among the four rectangles, the rectangle ADGI is the one for which one of the vertices lies in the third quadrant.
So, the vertices of the asked rectangle can be found as:
We know D(0,0) and A is at a distance of 5 units from the origin and lies on the negative x-axis. Therefore, we can say that A=(-5,0). Similarly, G is at a distance of 3 units from the origin and lies on the negative y-axis. So, G=(0,-3). And if we compare A and I, they will have their x- coordinate the same, which is -5 and also, G and I have the y-coordinate same, which is equal to -3. Therefore, we can say that I=(-5,-3).
Hence, the answer to the above question is option (c).
Note: You could have also answered the above question by easily eliminating the options, as the only option with one vertex in the third quadrant is option (c), but knowing the method is also important.
Complete step-by-step solution -
Now according to the question, one of the vertices is the origin, i.e., one of the vertices of the rectangle has coordinates (0,0). Now let us draw a diagram to check all the possible cases of rectangles which have one of their vertices to be the origin and the longer side of the rectangle lying along the x-axis.

Now among the four rectangles, the rectangle ADGI is the one for which one of the vertices lies in the third quadrant.
So, the vertices of the asked rectangle can be found as:
We know D(0,0) and A is at a distance of 5 units from the origin and lies on the negative x-axis. Therefore, we can say that A=(-5,0). Similarly, G is at a distance of 3 units from the origin and lies on the negative y-axis. So, G=(0,-3). And if we compare A and I, they will have their x- coordinate the same, which is -5 and also, G and I have the y-coordinate same, which is equal to -3. Therefore, we can say that I=(-5,-3).
Hence, the answer to the above question is option (c).
Note: You could have also answered the above question by easily eliminating the options, as the only option with one vertex in the third quadrant is option (c), but knowing the method is also important.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
EMI starts from ₹3,108.34 per month
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE




