
Write the relation between the angular momentum and torque. What is the torque acting on a body rotating with constant angular momentum?
Answer
439.2k+ views
Hint: We need to understand the physical quantities angular momentum and torque to understand the relation existing between them. We can find the definition for the angular momentum and the torque and easily solve the given problem.
Complete answer:
The angular momentum is the rotational analogue of the linear momentum and the torque is the rotational analogue of the force. The angular momentum is defined as the momentum imparted on a rotating object due to its perpendicular distance from the center of rotation. It is given as the cross product of the radius or the distance from the center of rotation to the center of mass of the object and the linear momentum.
i.e.,
Where, L is the angular momentum,
r is the distance from the center of mass to the axis of rotation,
p is the linear momentum,
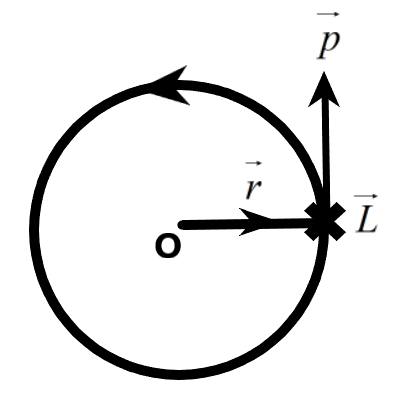
The torque on a rotating body is the force acting perpendicular to the distance between the axis of rotation. It provides the angular acceleration necessary for the object to undergo rotational motion. The torque is defined as the rate of change of angular momentum which is the same as the cross product of the linear force and the distance from the axis.
i.e.,
So, the torque is the rate of change of angular momentum. We are given that the angular momentum is constant for a situation. In such a situation the torque will be zero.
i.e.,
The torque will be zero in such a case. This is the required solution.
Note:
We can see that the force and the angular momentum are the same quantities as far as the rotational motion is concerned. But the net force acting on the system will not be equal to the angular momentum and therefore they are treated as different entities.
Complete answer:
The angular momentum is the rotational analogue of the linear momentum and the torque is the rotational analogue of the force. The angular momentum is defined as the momentum imparted on a rotating object due to its perpendicular distance from the center of rotation. It is given as the cross product of the radius or the distance from the center of rotation to the center of mass of the object and the linear momentum.
i.e.,
Where, L is the angular momentum,
r is the distance from the center of mass to the axis of rotation,
p is the linear momentum,

The torque on a rotating body is the force acting perpendicular to the distance between the axis of rotation. It provides the angular acceleration necessary for the object to undergo rotational motion. The torque is defined as the rate of change of angular momentum which is the same as the cross product of the linear force and the distance from the axis.
i.e.,
So, the torque is the rate of change of angular momentum. We are given that the angular momentum is constant for a situation. In such a situation the torque will be zero.
i.e.,
The torque will be zero in such a case. This is the required solution.
Note:
We can see that the force and the angular momentum are the same quantities as far as the rotational motion is concerned. But the net force acting on the system will not be equal to the angular momentum and therefore they are treated as different entities.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

Try out challenging quizzes on this topic
made by experts!
made by experts!
Take me there!



